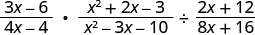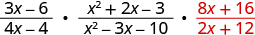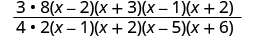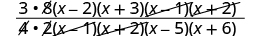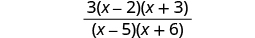Divide:
Solution
Got questions? Get instant answers now! Got questions? Get instant answers now!
If we have more than two rational expressions to work with, we still follow the same procedure. The first step will be to rewrite any division as multiplication by the reciprocal. Then we factor and multiply.
Key concepts
-
Multiplication of Rational Expressions
- If
are polynomials where
, then
.
- To multiply rational expressions, multiply the numerators and multiply the denominators
-
Multiply a Rational Expression
- Factor each numerator and denominator completely.
- Multiply the numerators and denominators.
- Simplify by dividing out common factors.
-
Division of Rational Expressions
- If
are polynomials where
, then
.
- To divide rational expressions multiply the first fraction by the reciprocal of the second.
-
Divide Rational Expressions
- Rewrite the division as the product of the first rational expression and the reciprocal of the second.
- Factor the numerators and denominators completely.
- Multiply the numerators and denominators together.
- Simplify by dividing out common factors.
Practice makes perfect
Multiply Rational Expressions
In the following exercises, multiply.
Divide Rational Expressions
In the following exercises, divide.
Everyday math
Probability The director of large company is interviewing applicants for two identical jobs. If
the number of women applicants and
the number of men applicants, then the probability that two women are selected for the jobs is
-
ⓐ Simplify the probability by multiplying the two rational expressions.
-
ⓑ Find the probability that two women are selected when
and
.
ⓐ
ⓑ
Got questions? Get instant answers now!
Area of a triangle The area of a triangle with base b and height h is
If the triangle is stretched to make a new triangle with base and height three times as much as in the original triangle, the area is
Calculate how the area of the new triangle compares to the area of the original triangle by dividing
by
.
Got questions? Get instant answers now!
Writing exercises
-
ⓐ Multiply
and explain all your steps.
-
ⓑ Multiply
and explain all your steps.
-
ⓒ Evaluate your answer to part (b) when
Did you get the same answer you got in part (a)? Why or why not?
Got questions? Get instant answers now!
-
ⓐ Divide
and explain all your steps.
-
ⓑ Divide
and explain all your steps.
-
ⓒ Evaluate your answer to part (b) when
Did you get the same answer you got in part (a)? Why or why not?
Got questions? Get instant answers now!
Self check
ⓐ After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section.
ⓑ After reviewing this checklist, what will you do to become confident for all objectives?