| << Chapter < Page | Chapter >> Page > |
Therefore is the time interval in the frame of reference where both events occur at the same location. It is the same interval of proper time discussed earlier. It also follows from the relation between and that that because is Lorentz invariant, the proper time is also Lorentz invariant. All observers in all inertial frames agree on the proper time intervals between the same two events.
Check Your Understanding Show that if a time increment dt elapses for an observer who sees the particle moving with velocity v , it corresponds to a proper time particle increment for the particle of
Start with the definition of the proper time increment:
where (
dx ,
dy ,
dx ,
cdt ) are measured in the inertial frame of an observer who does not necessarily see that particle at rest. This therefore becomes
We can deal with the difficulty of visualizing and sketching graphs in four dimensions by imagining the three spatial coordinates to be represented collectively by a horizontal axis, and the vertical axis to be the ct- axis. Starting with a particular event in space-time as the origin of the space-time graph shown, the world line of a particle that remains at rest at the initial location of the event at the origin then is the time axis. Any plane through the time axis parallel to the spatial axes contains all the events that are simultaneous with each other and with the intersection of the plane and the time axis, as seen in the rest frame of the event at the origin.
It is useful to picture a light cone on the graph, formed by the world lines of all light beams passing through the origin event A , as shown in [link] . The light cone, according to the postulates of relativity, has sides at an angle of if the time axis is measured in units of ct , and, according to the postulates of relativity, the light cone remains the same in all inertial frames. Because the event A is arbitrary, every point in the space-time diagram has a light cone associated with it.
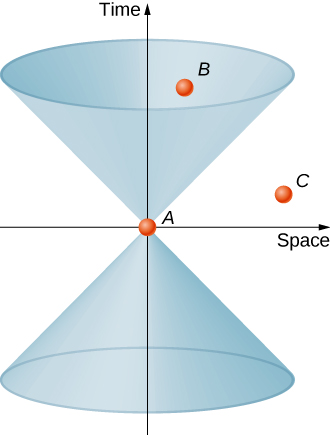
Consider now the world line of a particle through space-time. Any world line outside of the cone, such as one passing from A through C , would involve speeds greater than c , and would therefore not be possible. Events such as C that lie outside the light cone are said to have a space-like separation from event A . They are characterized by:
An event like B that lies in the upper cone is reachable without exceeding the speed of light in vacuum, and is characterized by
The event is said to have a time-like separation from A . Time-like events that fall into the upper half of the light cone occur at greater values of t than the time of the event A at the vertex and are in the future relative to A . Events that have time-like separation from A and fall in the lower half of the light cone are in the past, and can affect the event at the origin. The region outside the light cone is labeled as neither past nor future, but rather as “elsewhere.”

Notification Switch
Would you like to follow the 'University physics volume 3' conversation and receive update notifications?