2.3 Images formed by refraction  (Page 2/5)

Refraction at a convex surface

Consider a point source of light at point P in front of a convex surface made of glass (see [link] ). Let R be the radius of curvature, $n_1$ be the refractive index of the medium in which object point P is located, and $n_2$ be the refractive index of the medium with the spherical surface. We want to know what happens as a result of refraction at this interface.

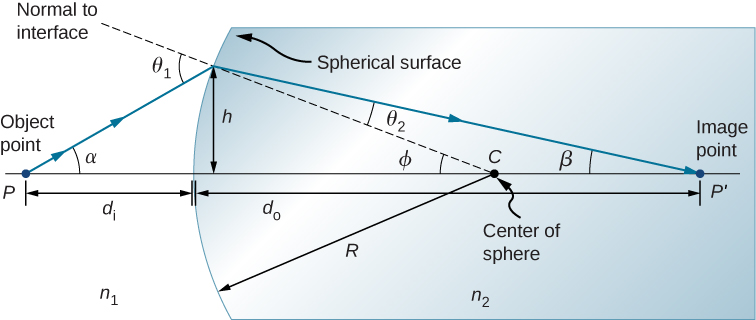
Because of the symmetry involved, it is sufficient to examine rays in only one plane. The figure shows a ray of light that starts at the object point P , refracts at the interface, and goes through the image point $P^{\prime }$ . We derive a formula relating the object distance $d_{\text{o}}$ , the image distance $d_{\text{i}}$ , and the radius of curvature R .

Applying Snell’s law to the ray emanating from point P gives $n_1\text{sin}{\theta }_1=n_2\text{sin}{\theta }_2$ . We work in the small-angle approximation, so $\text{sin}\theta \approx \theta$ and Snell’s law then takes the form

From the geometry of the figure, we see that

Inserting these expressions into Snell’s law gives

Using the diagram, we calculate the tangent of the angles $\alpha ,\beta ,\text{and}\varphi$ :

Again using the small-angle approximation, we find that $\text{tan}\theta \approx \theta$ , so the above relationships become

Putting these angles into Snell’s law gives

We can write this more conveniently as

If the object is placed at a special point called the first focus , or the object focus $F_1$ , then the image is formed at infinity, as shown in part (a) of [link] .

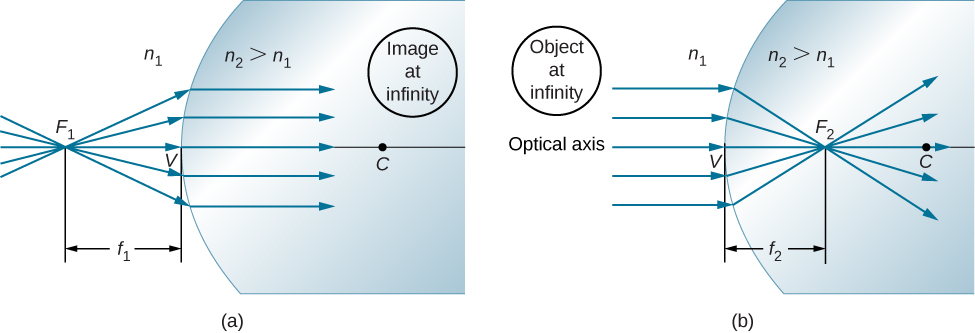
We can find the location $f_1$ of the first focus $F_1$ by setting $d_{\text{i}}=\text{∞}$ in the preceding equation.

Similarly, we can define a second focus or image focus $F_2$ where the image is formed for an object that is far away [part (b)]. The location of the second focus $F_2$ is obtained from [link] by setting $d_{\text{o}}=\text{∞}$ :

Note that the object focus is at a different distance from the vertex than the image focus because $n_1\ne n_2$ .

Sign convention for single refracting surfaces

Although we derived this equation for refraction at a convex surface, the same expression holds for a concave surface, provided we use the following sign convention:

1. $R>0$ if surface is convex toward object; otherwise, $R<0.$
2. $d_{\text{i}}>0$ if image is real and on opposite side from the object; otherwise, $d_{\text{i}}<0.$

Summary

This section explains how a single refracting interface forms images.

• When an object is observed through a plane interface between two media, then it appears at an apparent distance $h_{\text{i}}$ that differs from the actual distance $h_{\text{o}}$ : $h_{\text{i}}=(n_2\text{/}{n}_1)h_{\text{o}}$ .
• An image is formed by the refraction of light at a spherical interface between two media of indices of refraction $n_1$ and $n_2$ .
• Image distance depends on the radius of curvature of the interface, location of the object, and the indices of refraction of the media.

Conceptual questions

Problems