| << Chapter < Page | Chapter >> Page > |
Free body diagram
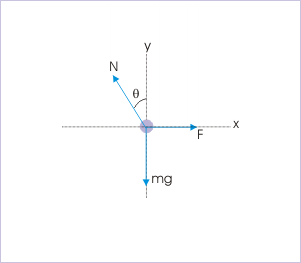
and
Taking ratio, we have :
Problem 4 : A string going over a pulley “A” of mass “m” supports a mass “M” as shown in the figure. Find the magnitude of force exerted by the clamp “B” on pulley “A”.
Balanced force system
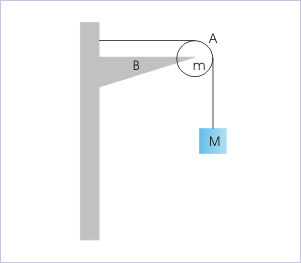
Solution : Here, we consider pulley as the body system. Let us also consider that clamp “B” exerts a force “F” in an arbitrary direction, making an angle with the horizontal. We should note that pulley, unless otherwise specified, is considered to be of negligible mass and friction-less. In this case, however, pulley has finite mass “m” and its weight should be considered to be an external force on the pulley.
The string is single piece and mass-less, whereas pulley is not mass-less. However, pulley is static. As such, there is no torque involved. Hence, tension in the string all through out is same. From the consideration of block, we see that tension in the string is equal to the weight of the block i.e. Mg.
Now, the external forces on pulley are (i) Horizontal tension "T" (ii) Weight, mg, of the pulley (iii) Vertical Tension, T, and (iv) force, F applied by clamp “B”.
Free body diagram

and
The force exerted by the clamp,F, is :
Problem 5 : Two blocks "A" and "B", weighing 20 N and 10 N respectively are in contact with each other. If the blocks are at rest, then find the force "F" and the normal reactions between all contact surfaces.
Balanced force system
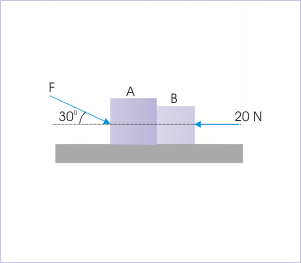
Solution : The question demands that we draw free body diagram of each of the block separately as we are required to know normal reactions at all surfaces. Here, there are three contact surfaces between (i) A and horizontal surface (ii) B and horizontal surface and (iii) A and B.
A preliminary assessment of forces on the blocks suggests that analysis of forces on B will provide values of unknown force(s). It is so because the forces on B are mutually perpendicular (thus, they would not need to be resolved), if appropriate coordinate system is chosen. Hence, we first analyze force on block B.
The external forces are (i) weight of B = 10 N (ii) Normal force applied by A i.e. (say) (iii) Normal force applied by surface i.e. and (iv) external force of 20 N
Free body diagram
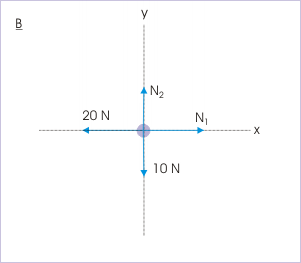
and
The external forces are (i) weight of A, 20 N (ii) Normal force applied by B i.e. (say) = = 20 N (iii) Normal force applied by surface i.e. and (iv) force, F = ?
Free body diagram
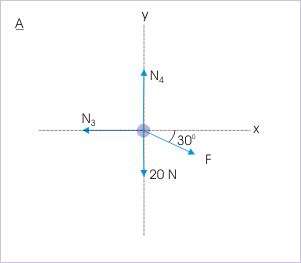
and
Problem 6 : The blocks A and B weighing 10 N and 20 N are connected by a string. The block B, in turn, is connected to block C with another string passing over a pulley. Friction forces at all interfaces is negligible. If the block system is in equilibrium, find the weight of C and tensions in the two strings.
Balanced force system
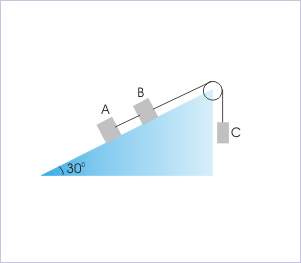
Solution : There are two strings. Hence, the tensions in the strings will be different. Let and be the tension in strings AB and BC respectively.
Looking at the various body systems, we guess that the simplest force system is the one associated with block C. However, force analysis of block C will not yield anything as we do not know its weight or the tension .
Thus, we begin with block A.
Free body diagram
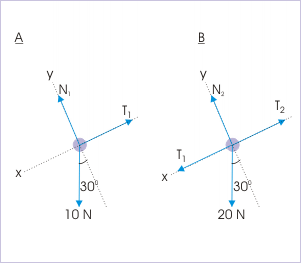
The external forces are (i) weight of A = 10 N (ii) Normal force applied by incline i.e. (say) (iii) tension in AB, .
We need not analyze forces in y – direction as we are not required to determine normal force and it is not expected to be used for analyzing force on block B.
The external forces are (i) weight of B, = 20 N (ii) Normal force applied by incline i.e. (say) (iii) tension in AB, and (iv) tension in BC, .
Free body diagram
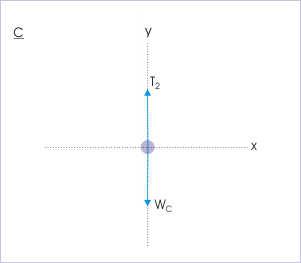
The external forces are (i) weight of c = ? and (ii) tension in BC, .
Author wishes to thank Scott Kravitz, Programming Assistant, Connexions for making suggestion to remove error in the module.

Notification Switch
Would you like to follow the 'Physics for k-12' conversation and receive update notifications?