8.9 Balanced force system

The motion under balanced force system is the subject matter of Newton's first law of motion. The condition of balanced force system simply implies that the net external force on the body is zero. This is exactly the situation for which Newton’s first law predicts that the body maintains its state of translational motion.

Mathematically, the net external force is zero for a balanced force system :

$$\sum F=0$$

This implies that linear acceleration of the point mass is zero,

$$a=0$$

For three dimensional rigid body, linear acceleration of the center of mass is zero (we shall discuss this aspect in detail subsequently in the course) ,

$$a_C=0$$

In component form,

$$\sum F_x=m{a}_x=0$$

$$\sum F_y=m{a}_y=0$$

$$\sum F_z=m{a}_z=0$$

It is to be noted here that the condition of zero net force does not mean necessarily “rest” as may be inferred from most of the examples in the immediate surrounding. A balanced force system simply indicates that velocity of the body does not change i.e.

$$v=\mathrm{constant}$$

Equilibrium

Equilibrium denotes a state of motion, which does not change due to external force or force system. Inversely, a force system, which does not change the state of motion of a body is said to be in equilibrium.

The state of motion of a body consists of both its translational and rotational states. A balanced force system, having zero net external force, does not guarantee equilibrium. A general consideration of equilibrium requires that force system should meet two conditions :

1. The net force is zero. In other words, force system is a balanced force system.
2. The net moment of force (torque) about any axis should be equal to zero.

Mathematically,

$$\sum F=0$$

$$\sum \tau =0$$

Equilibrium and balanced force system

It is clear that a balanced force system is a necessary condition for equilibrium, but it is not the sufficient condition to ensure equilibrium. However, a close look, at the body systems that we generally consider in dynamics, reveals that many of the systems eventually are restricted to translational motion.

There are following three situations under which a body is restricted to have only translational motion :

1: The body is an infinitesimally small entity to be approximated as a point. In this case, forces are concurrent, meeting at a common point.

2: The body is a real three dimensional entity. The forces on the body are concurrent at a common point. The common point, in turn, coincides with "center of mass" of the body.

3: The body is a real three dimensional entity. The forces on the body are not concurrent at a common point. However, the body is restrained to have only translational motion. The forces are though not concurrent, but we consider them concurrent at the "center of mass" of the body, as no rotational motion is involved.

In the nutshell, if we can treat the motion as translational motion without any angular or rotational motion involved, then a balanced force system with zero net force alone is sufficient to meet the requirement of equilibrium of the body.

Problem : A block of mass “m” is at rest on a rough incline of angle “θ”. Find the contact forces and the net contact force on the block.

A block on an incline

Solution : The forces on the block are (i) its weight (ii) normal force and (iii) Friction force. These forces are not concurrent (see figure).

Forces on the block

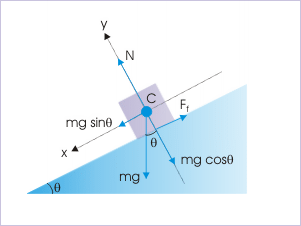
However, no turning effect is involved. We can, therefore, treat force system concurrent with the "center of mass" of the block. In order to analyze the forces, we consider a coordinate system as shown in the figure. Since the block is at rest, the forces on the block are balanced :

Free body diagram

$$\sum F_x=mg\sin \theta -F_f=0$$

$$\sum F_y=N-mg\cos \theta =0$$

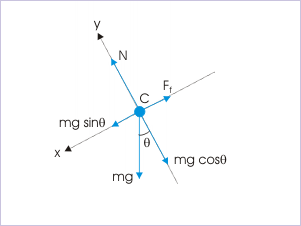
There are two contact forces (i) normal force and (ii) friction. The friction is given by the first equation :

$$F_f=mg\sin \theta$$

The normal force is given by the second equation :

$$N=mg\cos \theta$$

The net contact force is vector sum of two contact forces, which are at right angle to each other. Hence, magnitude of net contact force is :

$$F_C=\sqrt{\left(N^2+F_f^2\right)}$$

$$F_C=\sqrt{\left\{{\left(mg\right)}^2\left({\cos }^2\theta +{\sin }^2\theta \right)\right\}}$$

$$\Rightarrow F_C=mg$$

Got questions? Get instant answers now!

Coplanar force system

Though it has not been explicitly mentioned at any point in our study, but a scrutiny of the illustrations so far discussed indicates that most of the situations involve coplanar force systems. This simplifies force analysis greatly as we need to analyze force in two directions only :

$$\sum F_x=m{a}_x$$

$$\sum F_y=m{a}_y$$

If the force system is a balanced force system, then :

$$\sum F_x=m{a}_x=0$$

$$\sum F_y=m{a}_y=0$$

In case only translation is involved, then above two equations fulfills the requirement of the equilibrium as well.

Problem : : A body is subjected to four coplanar concurrent forces as shown in the figure. The body is under equilibrium. Find the forces “ $F_1$ ” and “ $F_2$ ”.

Four forces on a body

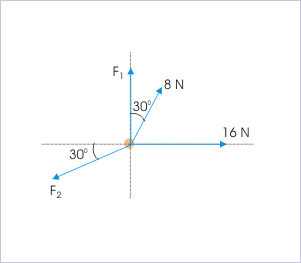
Solution : The body is in equilibrium under the action of coplanar concurrent forces. It means that forces form a balanced force system. We can treat the body as a point on the free body diagram. Let “x” and “y” be the axes of the coplanar coordinate system as shown in the figure. Then,

Free body diagram

$$\sum F_x=16+8\sin {30}^0-F_2\cos {30}^0=0$$

$$\sum F_y=F_1+8\cos {30}^0-F_2\sin {30}^0=0$$

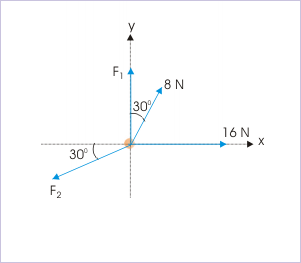
We can find “ $F_2$ ” from the first equation,

$$\Rightarrow F_{2}X\frac{\sqrt{3}}{2}=16+8X\frac{1}{2}=20$$

$$\Rightarrow F_2=20X\frac{2}{\sqrt{3}}=\frac{40}{\sqrt{3}}N$$

Substituting in second equation and solving for “ $F_1$ ”, we have :

$$\Rightarrow F_1=F_2\sin {30}^0-8\cos {30}^0=\frac{40}{\sqrt{3}}X\frac{1}{2}-8X\frac{\sqrt{3}}{2}$$

$$\Rightarrow F_1=\frac{20}{\sqrt{3}}-4X\sqrt{3}$$

$$\Rightarrow F_1=\frac{20-12}{\sqrt{3}}=\frac{8}{\sqrt{3}}N$$

Got questions? Get instant answers now!

Lami’s theorem

We have studied Lami’s theorem for three concurrent vectors (non-collinear vectors). If three concurrent forces are balanced, then

Lami's theorem

$$\frac{F_1}{\sin \alpha }=\frac{F_2}{\sin \beta }=\frac{F_3}{\sin \gamma }$$

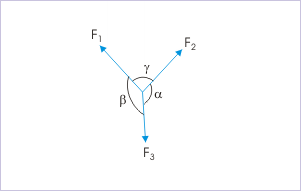
where α,β,γ are the angles between remaining two force not involved in the particular ratio. We can refer to the figure to understand the relation between forces and the angle involved. As net force is zero, forces represent three sides of a closed triangle. Clearly, three balanced concurrent forces are coplanar.

This theorem helps us to analyze balanced force system, comprising of exactly three concurrent coplanar forces.

Problem : A spherical mass “m” hanging from ceiling is displaced by applying a horizontal force,F. The string makes an angle “θ” with vertical as shown in the figure. Find force F.

Three forces on a spherical mass

Solution : Here, we shall employ Lami’s theorem as there are exactly three forces acting on the spherical mass. The FBD of the spherical mass is as shown in the figure.

Free body diagram

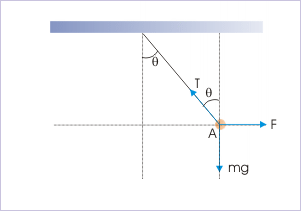
Applying Lami’s theorem, we have :

$$\frac{mg}{\sin \left({90}^0+\theta \right)}=\frac{T}{\sin {90}^0}=\frac{F}{\sin \left({180}^0-\theta \right)}$$

$$\Rightarrow \frac{mg}{\cos \theta }=\frac{T}{1}=\frac{F}{\sin \theta }$$

From the last two ratios, we have :

$$\Rightarrow F=T\sin \theta$$

From the first two ratios, we have :

$$\Rightarrow T=\frac{mg}{\cos \theta }$$

Combining two equations,

$$\Rightarrow F=\frac{mg\sin \theta }{\cos \theta }=mg\tan \theta$$

Lami's theroem provides an alternative to normal component analysis - may be with some computational advantage. Nevertheless, we should keep in mind that it is just an alternative method. We could have solved this problem, using component analysis as :

$$\begin{array}{l}\sum F_x=F-T\sin \theta =0\\ \Rightarrow F=T\sin \theta \end{array}$$

and

$$\begin{array}{l}\sum F_y=\mathrm{mg}-T\cos \theta =0\\ \Rightarrow \mathrm{mg}=T\cos \theta \end{array}$$

Taking ratio to eliminate “T”,

$$\begin{array}{l}\Rightarrow \frac{F}{\mathrm{mg}}=\tan \theta \\ \Rightarrow F=\mathrm{mg}\tan \theta \end{array}$$

Got questions? Get instant answers now!