18.1 Torque  (Page 3/3)

Relative orientation of the plane of operand vectors

There are two possibilities here. The plane of operands are same as that of the plane of coordinate system. In simple word, the plane of velocity and position vector is same as one of three planes formed by coordinate system. If this is so, then problem analysis is greatly simplified. Clearly, the torque will be along the remaining third axis. We have only to find the orientation (in the positive or negative direction of the axis) with the help of right hand rule. It is evident that we should always strive to orient our coordinate system, if possible, so that the plane formed by position vector and force align with one of the coordinate planes.

In case, the two planes are different, then we need to be careful (i) in first identifying the plane of operands (ii) finding relation of the operands plane with coordinate plane and (iii) applying right hand rule to determine orientation (which side of the plane). Importantly, we can not treat torque as scalar with appropriate sign, but have to find direction of torque with respect to one of the coordinates.

Problem : A particle in xy plane is acted by a force 5 N in z-direction as shown in the figure. Find the net torque on the particle.

Torque on a particle

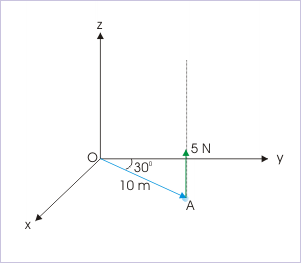
Solution : In this case, moment arm is equal to the magnitude of position vector. Hence, torque is :

$$\begin{array}{l}\tau =10x5=50Nm\end{array}$$

However, finding its direction is not as straight forward. The situation here differs to earlier examples in one important respect. The plane containing position vector and force vector is a plane defined by zOA. Also, this plane is perpendicular to xy - plane. Now, torque is passing through the origin and is perpendicular to the plane zoA. We know a theorem of geometry that angles between two lines and their perpendiculars are same. Applying the same and right hand rule, we conclude that the torque is shifted by 30 degree from the y-axis and lies in xy-plane as shown in the figure here.

Torque on a particle

Got questions? Get instant answers now!

Torque in component form

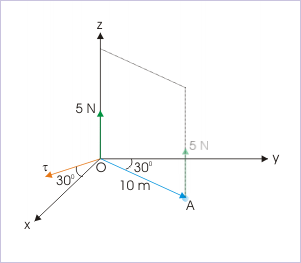
Torque, being a vector, can be evaluated in component form with the help of unit vectors along the coordinate axes. The various expressions involved in the vector algebraic analysis are as given here :

$$\begin{array}{l}\mathbf{\tau }=\mathbf{r}\mathbf{x}\mathbf{F}\end{array}$$

$$\begin{array}{l}\mathbf{\tau }=(x\mathbf{i}+y\mathbf{j}+z\mathbf{k})\mathbf{x}(F_x\mathbf{i}+F_y\mathbf{j}+F_z\mathbf{k})\end{array}$$

τ = | i j k | | x y z || Fx Fy Fz|

Summary

1: Torque in general angular motion is defined with respect to a point as against axis in rotation. The expression of torque in two cases, however, is same :

$$\begin{array}{l}\mathbf{\tau }=\mathbf{r}\mathbf{x}\mathbf{F}\end{array}$$

2: When the point (about which torque is defined) coincides with the origin of coordinate system, the vector “ r ” appearing in the expression of torque is position vector.

3: Magnitude of torque is given by either of the following relations,

(i) Torque in terms of angle enclosed

$$\begin{array}{l}\tau =rF\sin \theta \end{array}$$

(ii) Torque in terms of force perpendicular to position vector

$$\begin{array}{l}\tau =r{F}_{\perp }\end{array}$$

(iii) Torque in terms of moment arm

$$\begin{array}{l}\tau =r_{\perp }F\end{array}$$

4: Direction of torque

The torque is perpendicular to the plane formed by velocity vector and force and also individually to either of them. We get to know the orientation of the torque vector by applying right hand rule.

5: When more than one force acts, then we should determine the resultant of forces or resultant of torques, depending on which of the approach will minimize or avoid non-linear vector summation to obtain the result.

6: When the plane of operands is same as one of the coordinate plane, then torque acts along the remaining axis.

7: Torque is expressed in component form as :

τ = | i j k | | x y z || Fx Fy Fz|