8.2 Newton's second law of motion

In mathematical terms,

For invariant mass (the mass of the body under consideration does not change during application of force), we can take out mass "m" from the differential sign :

As mass “m” is a positive scalar quantity, directions of force and acceleration are same. The dimensional formula of force is $\left[ML{T}^{-2}\right]$ and its SI unit of measurement is Newton, which is equal to " $kg-m∕s^2$ ". One Newton (denoted by symbol "N") is defined as the force, which when applied on a point mass of 1 kg produces an acceleration of 1 $m∕s^2$ .

Example

Problem 1 : A ball, weighing 10 gm, hits a hard surface in normal direction with a speed of 10 m/s and rebounds with the same speed. The ball remains in contact with the hard surface for 0.1 s. Find the magnitude of average force on the ball, applied by the surface.

Solution : We can find average force as the product of mass and average acceleration during the contact. Average acceleration is :

$$\begin{array}{l}{a}_{\mathrm{avg}}=\frac{\Delta v}{\Delta t}=\frac{v_2-v_1}{\Delta t}\end{array}$$

If the rebound direction is considered positive, then $v_1$ = -10 m/s and $v_2$ = 10 m/s.

$$\begin{array}{l}\Rightarrow a_{\mathrm{avg}}=\frac{10-(-10)}{0.1}=200m/s^2\end{array}$$

The average force is :

$$\begin{array}{l}\Rightarrow F_{\mathrm{avg}}=m{a}_{\mathrm{avg}}=10X200=2000N\end{array}$$

Interpreting newton's second law of motion

Few important aspects of Newton’s second law of motion are discussed in the following sections :

Deduction of first law of motion

Newton's first law of motion is a subset of second law in the sense that first law is just a specific description of motion, when net external force on the body is zero.

$$\mathbf{F}=m\mathbf{a}=0$$

This means that if there is no net external force on the body, then acceleration of the body is zero. Equivalently, we can state that if there is no net external force on the body, then velocity of the body can not change. Further, it is also easy to infer that if there is no net external force on the body, it will maintain its state of motion. These are exactly the statements in which first law of motion are stated.

Forces on the body

The body under investigation may be acted upon by a number of forces. We must use vector sum of all external forces, while applying second law of motion. A more general form of Second law of motion valid for a system of force is :

The vector addition of forces as required in the left hand side of the equation excludes the forces that the body applies on other bodies. We shall know from Third law of motion that force always exists in the pair of “action” and “reaction”. Hence, if there are “n” numbers of forces acting on the body, then there are “n” numbers of forces that the body exerts on other bodies. We must carefully exclude all such forces that body as a reaction applies on other bodies.

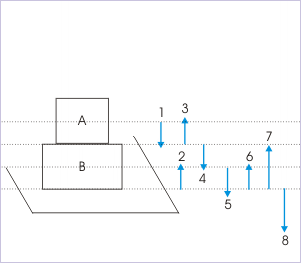
Consider two blocks “A” and “B” lying on horizontal surface as shown in the figure. There are many forces in action here :

Forces acting on two blocks and earth

1. Earth pulls down “A” (force of gravitation)
2. “A” pulls up Earth (force of gravitation)
3. “B” pushes up “A” (normal force)
4. “A” pushes down “B” (weight of “A” : normal force)
5. Earth pulls down “B” (force of gravitation)
6. “B” pulls up Earth (force of gravitation)
7. Earth pushes up “B” (normal force) and
8. “B” pushes down the Earth (weight of “B” and "A" : normal force)