Definitions, simple applications, and graphs of trigonometric  (Page 6/7)

$\theta$	0 ${}^{\circ }$	30 ${}^{\circ }$	60 ${}^{\circ }$	90 ${}^{\circ }$	120 ${}^{\circ }$	150 ${}^{\circ }$
$cos\theta$
$\theta$	180 ${}^{\circ }$	210 ${}^{\circ }$	240 ${}^{\circ }$	270 ${}^{\circ }$	300 ${}^{\circ }$	330 ${}^{\circ }$	360 ${}^{\circ }$
$cos\theta$

Let us look back at our values for $cos\theta$

If you look carefully, you will notice that the cosine of an angle $\theta$ is the same as the sine of the angle ${90}^{\circ }-\theta$ . Take for example,

This tells us that in order to create the cosine graph, all we need to do is to shift the sine graph ${90}^{\circ }$ to the left. The graph of $cos\theta$ is shown in [link] . As the cosine graph is simply a shifted sine graph, it will have the same period and amplitude as the sine graph.

Functions of the form $y=acos\left(x\right)+q$

In the equation, $y=acos\left(x\right)+q$ , $a$ and $q$ are constants and have different effects on the graph of the function. The general shape of the graph of functions of this form is shown in [link] for the function $f\left(\theta \right)=2cos\theta +3$ .

Functions of the form $y=acos\left(\theta \right)+q$ :

1. On the same set of axes, plot the following graphs:
   1. $a\left(\theta \right)=cos\theta -2$
   2. $b\left(\theta \right)=cos\theta -1$
   3. $c\left(\theta \right)=cos\theta$
   4. $d\left(\theta \right)=cos\theta +1$
   5. $e\left(\theta \right)=cos\theta +2$
   Use your results to deduce the effect of $q$ .
2. On the same set of axes, plot the following graphs:
   1. $f\left(\theta \right)=-2·cos\theta$
   2. $g\left(\theta \right)=-1·cos\theta$
   3. $h\left(\theta \right)=0·cos\theta$
   4. $j\left(\theta \right)=1·cos\theta$
   5. $k\left(\theta \right)=2·cos\theta$
   Use your results to deduce the effect of $a$ .

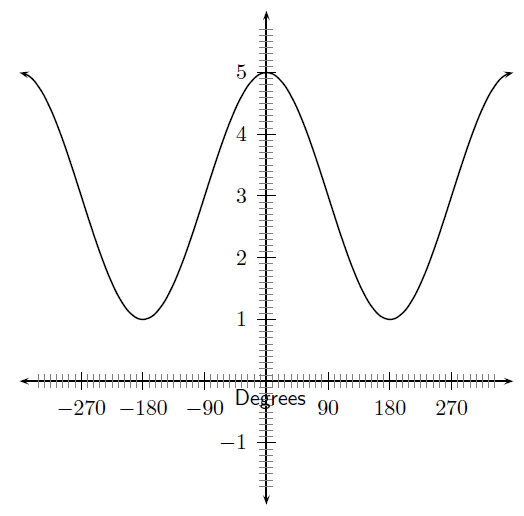
You should have found that the value of $a$ affects the amplitude of the cosine graph in the same way it did for the sine graph.

You should have also found that the value of $q$ shifts the cosine graph in the same way as it did the sine graph.

These different properties are summarised in [link] .

	$a>0$	$a<0$
$q>0$
$q<0$

Domain and range

For $f\left(\theta \right)=acos\left(\theta \right)+q$ , the domain is $\{\theta :\theta \in \mathbb{R}\}$ because there is no value of $\theta \in \mathbb{R}$ for which $f\left(\theta \right)$ is undefined.

It is easy to see that the range of $f\left(\theta \right)$ will be the same as the range of $asin\left(\theta \right)+q$ . This is because the maximum and minimum values of $acos\left(\theta \right)+q$ will be the same as the maximum and minimum values of $asin\left(\theta \right)+q$ .

Intercepts

The $y$ -intercept of $f\left(\theta \right)=acos\left(x\right)+q$ is calculated in the same way as for sine.

Comparison of graphs of $sin\theta$ And $cos\theta$

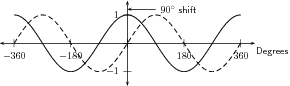
Notice that the two graphs look very similar. Both oscillate up and down around the $x$ -axis as you move along the axis. The distances between the peaks of the two graphs is the same and is constant along each graph. The height of the peaks and the depths of the troughs are the same.

The only difference is that the $sin$ graph is shifted a little to the right of the $cos$ graph by 90 ${}^{\circ }$ . That means that if you shift the whole $cos$ graph to the right by 90 ${}^{\circ }$ it will overlap perfectly with the $sin$ graph. You could also move the $sin$ graph by 90 ${}^{\circ }$ to the left and it would overlap perfectly with the $cos$ graph. This means that:

Graph of $tan\theta$

Graph of $tan\theta$

Complete the following table, using your calculator to calculate the values correct to 1 decimal place. Then plot the values with $tan\theta$ on the $y$ -axis and $\theta$ on the $x$ -axis.

$\theta$	0 ${}^{\circ }$	30 ${}^{\circ }$	60 ${}^{\circ }$	90 ${}^{\circ }$	120 ${}^{\circ }$	150 ${}^{\circ }$
$tan\theta$
$\theta$	180 ${}^{\circ }$	210 ${}^{\circ }$	240 ${}^{\circ }$	270 ${}^{\circ }$	300 ${}^{\circ }$	330 ${}^{\circ }$	360 ${}^{\circ }$
$tan\theta$