6.8 Quantum numbers and rules  (Page 3/10)

where $s$ is defined to be the spin quantum number    . This is very similar to the quantization of $L$ given in $L=\sqrt{l\left(l+1\right)}\frac{h}{\mathrm{2\pi }}$ , except that the only value allowed for $s$ for electrons is 1/2.

The direction of intrinsic spin is quantized , just as is the direction of orbital angular momentum. The direction of spin angular momentum along one direction in space, again called the $z$ -axis, can have only the values

for electrons. $S_z$ is the $z$ -component of spin angular momentum and $m_s$ is the spin projection quantum number    . For electrons, $s$ can only be 1/2, and $m_s$ can be either +1/2 or –1/2. Spin projection $m_s\text{=+}1/2$ is referred to as spin up , whereas $m_s=-1/2$ is called spin down . These are illustrated in [link] .

To summarize, the state of a system, such as the precise nature of an electron in an atom, is determined by its particular quantum numbers. These are expressed in the form $\left(\mathrm{n,\; l,}{m}_l,m_s\right)$ —see [link] For electrons in atoms , the principal quantum number can have the values $n=\mathrm{1,\; 2,\; 3,\; ...}$ . Once $n$ is known, the values of the angular momentum quantum number are limited to $l=\mathrm{1,\; 2,\; 3,\; ...},n-1$ . For a given value of $l$ , the angular momentum projection quantum number can have only the values $m_l=-l,-l+\mathrm{1,\; ...},-\mathrm{1,\; 0,\; 1,\; ...},l-\mathrm{1,}l$ . Electron spin is independent of $\mathrm{n,\; l,}$ and $m_l$ , always having $s=1/2$ . The spin projection quantum number can have two values, $m_s=1/2\text{or}-1/2$ .

[link] shows several hydrogen states corresponding to different sets of quantum numbers. Note that these clouds of probability are the locations of electrons as determined by making repeated measurements—each measurement finds the electron in a definite location, with a greater chance of finding the electron in some places rather than others. With repeated measurements, the pattern of probability shown in the figure emerges. The clouds of probability do not look like nor do they correspond to classical orbits. The uncertainty principle actually prevents us and nature from knowing how the electron gets from one place to another, and so an orbit really does not exist as such. Nature on a small scale is again much different from that on the large scale.

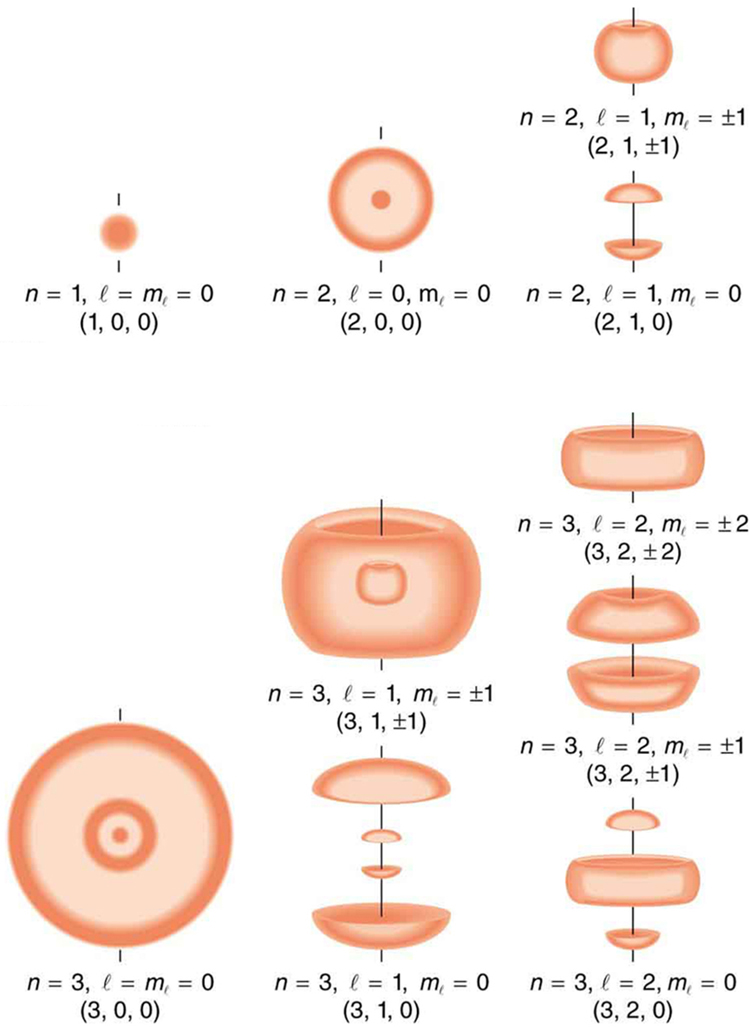
We will see that the quantum numbers discussed in this section are valid for a broad range of particles and other systems, such as nuclei. Some quantum numbers, such as intrinsic spin, are related to fundamental classifications of subatomic particles, and they obey laws that will give us further insight into the substructure of matter and its interactions.

Phet explorations: stern-gerlach experiment

The classic Stern-Gerlach Experiment shows that atoms have a property called spin. Spin is a kind of intrinsic angular momentum, which has no classical counterpart. When the z-component of the spin is measured, one always gets one of two values: spin up or spin down.

Section summary

• Quantum numbers are used to express the allowed values of quantized entities. The principal quantum number $n$ labels the basic states of a system and is given by
  $n=\mathrm{1,}\mathrm{2,}\mathrm{3,}\text{.}\text{.}\text{.}.$
• The magnitude of angular momentum is given by
  $L=\sqrt{l\left(l+1\right)}\frac{h}{\mathrm{2\pi }}\left(l=\mathrm{0,\; 1,\; 2,\; ...},n-1\right),$
  where $l$ is the angular momentum quantum number. The direction of angular momentum is quantized, in that its component along an axis defined by a magnetic field, called the $z$ -axis is given by
  $L_z=m_l\frac{h}{\mathrm{2\pi }}\left(m_l=-l,-l+\mathrm{1,\; ...},-\mathrm{1,\; 0,\; 1,\; ...}l-\mathrm{1,}l\right)\text{,}$
  where $L_z$ is the $z$ -component of the angular momentum and $m_l$ is the angular momentum projection quantum number. Similarly, the electron’s intrinsic spin angular momentum $S$ is given by
  $S=\sqrt{s\left(s+1\right)}\frac{h}{\mathrm{2\pi }}\text{(}s=1/2\text{for electrons),}$
  $s$ is defined to be the spin quantum number. Finally, the direction of the electron’s spin along the $z$ -axis is given by
  $S_z=m_s\frac{h}{\mathrm{2\pi }}\left(m_s=-\frac{1}{2},+\frac{1}{2}\right)\text{,}$
  where $S_z$ is the $z$ -component of spin angular momentum and $m_s$ is the spin projection quantum number. Spin projection $m_s\text{=+}1/2$ is referred to as spin up, whereas $m_s=-1/2$ is called spin down. [link] summarizes the atomic quantum numbers and their allowed values.

Conceptual questions

Problem exercises