| << Chapter < Page | Chapter >> Page > |
Because r is significant and the scatter plot shows a linear trend, the regression line can be used to predict final exam scores.
The 95% Critical Values of the Sample Correlation Coefficient Table can be used to give you a good idea of whether the computed value of is significant or not . Compare r to the appropriate critical value in the table. If r is not between the positive and negative critical values, then the correlation coefficient is significant. If r is significant, then you may want to use the line for prediction.
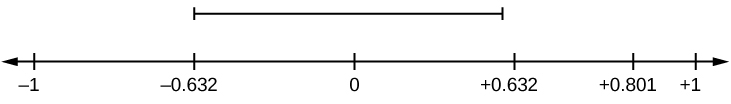
Suppose you computed r = 0.801 using n = 10 data points. df = n - 2 = 10 - 2 = 8. The critical values associated with df = 8 are -0.632 and + 0.632. If r <negative critical value or r >positive critical value, then r issignificant. Since r = 0.801 and 0.801>0.632, r is significant and the line may be usedfor prediction. If you view this example on a number line, it will help you.
For a given line of best fit, you computed that r = 0.6501 using n = 12 data points and the critical value is 0.576. Can the line be used for prediction? Why or why not?
If the scatter plot looks linear then, yes, the line can be used for prediction, because r >the positive critical value.
Suppose you computed r = –0.624 with 14 data points. df = 14 – 2 = 12. The critical values are –0.532 and 0.532. Since –0.624<–0.532, r is significant and the line can be used for prediction

For a given line of best fit, you compute that r = 0.5204 using n = 9 data points, and the critical value is 0.666. Can the line be used for prediction? Why or why not?
No, the line cannot be used for prediction, because r <the positive critical value.
Suppose you computed r = 0.776 and n = 6. df = 6 – 2 = 4. The critical values are –0.811 and 0.811. Since –0.811<0.776<0.811, r is not significant, and the line should not be used for prediction.

For a given line of best fit, you compute that r = –0.7204 using n = 8 data points, and the critical value is = 0.707. Can the line be used for prediction? Why or why not?
Yes, the line can be used for prediction, because r<the negative critical value.
Consider the third exam/final exam example . The line of best fit is: ŷ = –173.51+4.83 x with r = 0.6631 and there are n = 11 data points. Can the regression line be used for prediction? Given a third-exam score ( x value), can we use the line to predict the final exam score (predicted y value)?

Notification Switch
Would you like to follow the 'Introductory statistics fall 2014' conversation and receive update notifications?