11.2 Test of a single variance  (Page 2/22)

Professor Price has a weakness for cream filled donuts, but he believes that some bakeries are not properly filling the donuts. A sample of 24 donuts reveals a meanamount of filling equal to 0.5 cups, and the sample standard deviation is 0.11 cups. Professor Price has an interest in the average quantity of filling, of course, but he isparticularly distressed if one donut is radically different from another. Professor Price does not like surprises.

Try it

The FCC conducts broadband speed tests to measure how much data per second passes between a consumer’s computer and the internet. As of August of 2012, the standard deviation of Internet speeds across Internet Service Providers (ISPs) was 12.2 percent. Suppose a sample of 15 ISPs is taken, and the standard deviation is 13.2. An analyst claims that the standard deviation of speeds is more than what was reported. State the null and alternative hypotheses, compute the degrees of freedom, the test statistic, sketch the graph of the distribution and mark the area associated with the level of confidence, and draw a conclusion. Test at the 1% significance level.

H ₀ : σ ² = 12.2 ²

H _a : σ ² >12.2 ²
df = 14
chi ² test statistic = 16.39

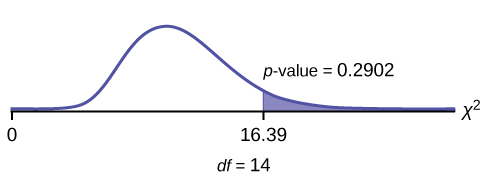
The p -value is 0.2902, so we decline to reject the null hypothesis. There is not enough evidence to suggest that the variance is greater than 12.2 ² .

In 2nd DISTR , use7: χ2cdf . The syntax is (lower, upper, df) for the parameter list. χ2cdf(16.39,10^99,14) . The p -value = 0.2902.

References

“AppleInsider Price Guides.” Apple Insider, 2013. Available online at http://appleinsider.com/mac_price_guide (accessed May 14, 2013).

Data from the World Bank, June 5, 2012.

Chapter review

To test variability, use the chi-square test of a single variance. The test may be left-, right-, or two-tailed, and its hypotheses are always expressed in terms of the variance (or standard deviation).

Formula review

${\chi }^2=$ $\frac{(n-1)s^2}{{\sigma }_0^2}$ Test of a single variance statistic where:
n : sample size
s : sample standard deviation
${\sigma }_0$ : hypothesized value of the population standard deviation

df = n – 1 Degrees of freedom

Test of a single variance

• Use the test to determine variation.
• The degrees of freedom is the number of samples – 1.
• The test statistic is $\frac{(n–1)s^2}{{\sigma }_0^2}$ , where n = the total number of data, s ² = sample variance, and σ ² = population variance.
• The test may be left-, right-, or two-tailed.

Use the following information to answer the next three exercises: An archer’s standard deviation for his hits is six (data is measured in distance from the center of the target). An observer claims the standard deviation is less.

Use the following information to answer the next three exercises: The standard deviation of heights for students in a school is 0.81. A random sample of 50 students is taken, and the standard deviation of heights of the sample is 0.96. A researcher in charge of the study believes the standard deviation of heights for the school is greater than 0.81.

Use the following information to answer the next four exercises: The average waiting time in a doctor’s office varies. The standard deviation of waiting times in a doctor’s office is 3.4 minutes. A random sample of 30 patients in the doctor’s office has a standard deviation of waiting times of 4.1 minutes. One doctor believes the variance of waiting times is greater than originally thought.