Points, lines and angles  (Page 3/3)

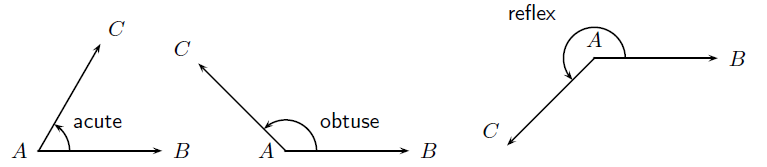
Once angles can be measured, they can then be compared. For example, all right angles are 90 ${}^{\circ }$ , therefore all right angles are equal and an obtuse angle will always be larger than an acute angle.

The following video summarizes what you have learnt so far about angles.

Special angle pairs

In [link] , straight lines $AB$ and $CD$ intersect at point X, forming four angles: $\widehat{X_1}$ or $\angle BXD$ , $\widehat{X_2}$ or $\angle BXC$ , $\widehat{X_3}$ or $\angle CXA$ and $\widehat{X_4}$ or $\angle AXD$ .

The table summarises the special angle pairs that result.

The following video summarises what you have learnt so far

Parallel lines intersected by transversal lines

Two lines intersect if they cross each other at a point. For example, at a traffic intersection two or more streets intersect; the middle of the intersection is the common point between the streets.

Parallel lines are lines that never intersect. For example the tracks of a railway line are parallel (for convenience, sometimes mathematicians say they intersect at 'a point at infinity', i.e. an infinite distance away). We wouldn't want the tracks to intersect after as that would be catastrophic for the train!

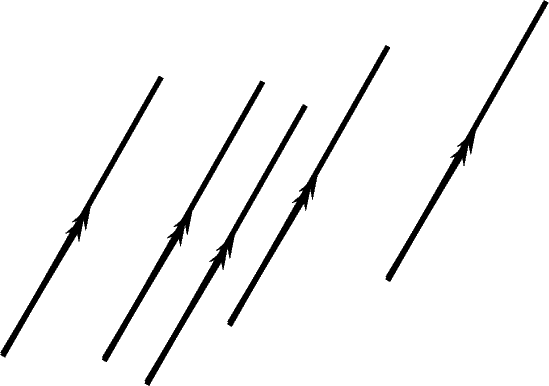
All these lines are parallel to each other. Notice the pair of arrow symbols for parallel.

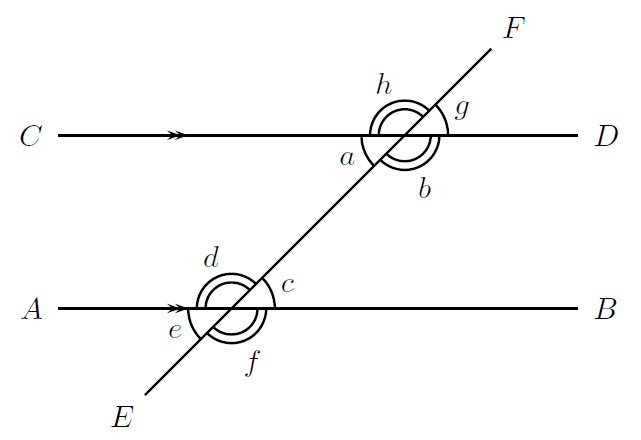
A transversal of two or more lines is a line that intersects these lines. For example in [link] , $AB$ and $CD$ are two parallel lines and $EF$ is a transversal. We say $AB\parallel CD$ . The properties of the angles formed by these intersecting lines are summarised in the table below.

Name of angle	Definition	Examples	Notes
interior angles	the angles that lie inside the parallel lines	in [link] $a$ , $b$ , $c$ and $d$ are interior angles	the word interior means inside
adjacent angles	the angles share a common vertex point and line	in [link] ( $a$ , $h$ ) are adjacent and so are ( $h$ , $g$ ); ( $g$ , $b$ ); ( $b$ , $a$ )
exterior angles	the angles that lie outside the parallel lines	in [link] $e$ , $f$ , $g$ and $h$ are exterior angles	the word exterior means outside
alternate interior angles	the interior angles that lie on opposite sides of the transversal	in [link] ( $a,c$ ) and ( $b$ , $d$ ) are pairs of alternate interior angles, $a=c$ , $b=d$
co-interior angles on the same side	co-interior angles that lie on the same side of the transversal	in [link] ( $a$ , $d$ ) and ( $b$ , $c$ ) are interior angles on the same side. $a+d={180}^{\circ }$ , $b+c={180}^{\circ }$
corresponding angles	the angles on the same side of the transversal and the same side of the parallel lines	in [link] $(a,e)$ , $(b,f)$ , $(c,g)$ and $(d,h)$ are pairs of corresponding angles. $a=e$ , $b=f$ , $c=g$ , $d=h$

The following video summarises what you have learnt so far

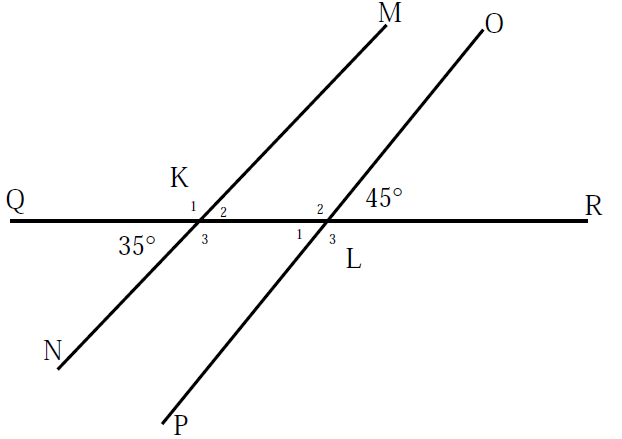
Find all the unknown angles in the following figure:

1. Find x $\text{AB}\parallel \text{CD}$ . So $x={30}^{°}$ (alternate interior angles)
2. Find y
   $\begin{array}{ccc}\hfill 160+y& =& 180\hfill \\ \hfill y& =& {20}^{°}\hfill \end{array}$
   (co-interior angles on the same side)

Got questions? Get instant answers now!

Determine if there are any parallel lines in the following figure:

1. Decide which lines may be parallel Line EF cannot be parallel to either AB or CD since it cuts both these lines. Lines AB and CD may be parallel.
2. Determine if these lines are parallel We can show that two lines are parallel if we can find one of the pairs of special angles. We know that ${\hat{E}}_2={25}^{°}$ (opposite angles). And then we note that
   $\begin{array}{ccc}\hfill {\hat{E}}_2& =& {\hat{F}}_4\hfill \\ & =& {25}^{°}\hfill \end{array}$
   So we have shown that $\text{AB}\parallel \text{CD}$ (corresponding angles)

Got questions? Get instant answers now!

Angles

1. Use adjacent, corresponding, co-interior and alternate angles to fill in all the angles labeled with letters in the diagram below:

   

2. Find all the unknown angles in the figure below:

   

3. Find the value of $x$ in the figure below:

   

4. Determine whether there are pairs of parallel lines in the following figures.

   1. 

   2. 

   3. 

5. If AB is parallel to CD and AB is parallel to EF, prove that CD is parallel to EF:

   

The following video shows some problems with their solutions