| << Chapter < Page | Chapter >> Page > |
F = ma
The second Physics concept we need has to do with force. When a force is applied to an object, the object will accelerate unless there is another equal force to offset it. The acceleration is measured as a change in either the speed of the object or its direction or both. The amount of acceleration depends on the mass of the object: the heavier the object, the less it accelerates. Newton’s law tells us that the force F applied to an object is equal to the acceleration of the object a times its mass m :
Using these two equations, we will be able to relate the pressure of a gas to the mass of the gas particles and their acceleration when they hit the walls of the container. This means that, in principle, we should be able to find the pressure of a gas from the properties and movements of the gas particles. However, in a typical sample of a gas there might be a mole or many moles of particles. Understanding the motions of 10 23 or more molecules will require some interesting observations and some very careful thinking about how to interpret these observations.
One of the amazing things about the Ideal Gas Law is that it predicts exactly the same pressure for every type of gas. If we know the temperature of the gas, the volume it is contained in, and the number of moles of the gas, then we can predict its pressure without even knowing what type of gas we have. This is very surprising. We know about the structure and bonding of molecules and we know some of the properties of molecules that the structure and bonding create. These properties vary a lot from one molecule to the next. In the Foundations, we learned that the pressure of a gas must be somehow related to the movement of the gas particles. It would seem that different molecules would move differently and this would cause different pressures. But this is not the case.
This suggests that we should look for experimental conditions where the pressures of different gases are different. In other words, we need to find conditions where the predictions of the Ideal Gas Law aren’t correct. If we can find such conditions, we would find that P is no longer equal to nRT/V . One way to do this is to plot P versus nRT/V for a gas: we can vary n , V , and T and look at how P changes. Rather than vary all three variables at the same time, we’ll take a fixed T and vary the “particle density,” n/V . We can increase n/V by either pumping in more gas to increase n or by decreasing the volume of the container. Either way, we only have one variable to look at, which is the density of particles in the container. If we plot P versus nRT/V , we should get a straight line, since the Ideal Gas Law predicts that these are always equal to each other. This straight line should be exactly the same for all gases under all conditions if the Ideal Gas Law is valid. The results of such an experiment are shown in Figure 1 for three different gases.
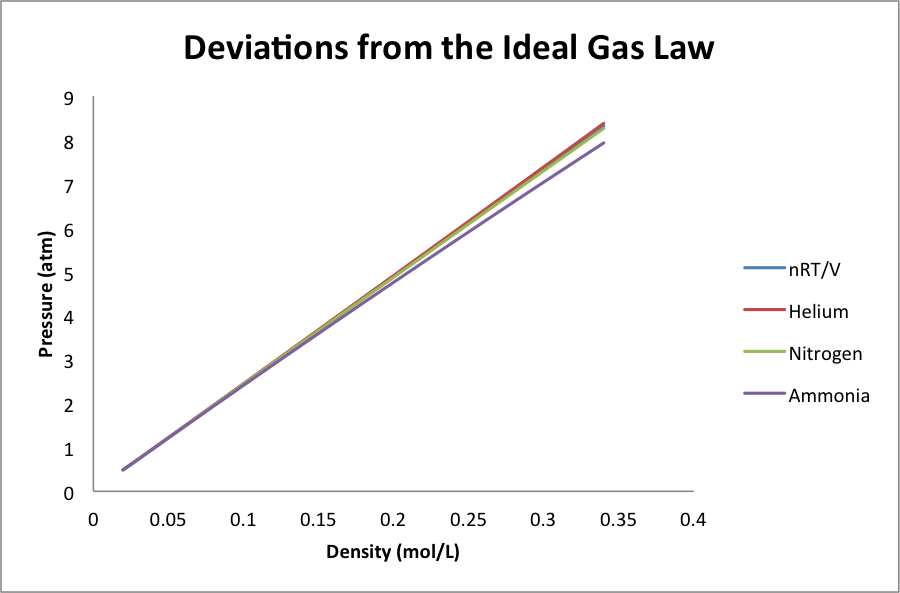
These data show two features which should catch your eye. The first is that all three graphs look very much like the straight line predicted by the Ideal Gas Law even when we increase the density of particles by a factor of 10. Therefore, the Ideal Gas Law works really well even when we increase the density of the gas by quite a lot. The second feature, however, is that if we look very closely at the three graphs for the three different gases at the highest density, we see that the pressures start to vary away from the Ideal Gas Law and away from each other. This is a little hard to see in Figure 1, so we’ll try plotting the data more clearly in Figure 2. This time, we will plot the ratio PV/nRT versus n/V . If the Ideal Gas Law works, then PV/nRT should always be equal to 1. If not, PV/nRT will be something different than 1, either higher or lower. This means that measuring PV/nRT in an experiment is a way for us to tell whether the Ideal Gas Law is valid.

Notification Switch
Would you like to follow the 'Concept development studies in chemistry 2013' conversation and receive update notifications?