Points, lines and angles

Introduction

The purpose of this chapter is to recap some of the ideas that you learned in geometry and trigonometry in earlier grades. You should feel comfortable with the work covered in this chapter before attempting to move onto the Grade 10 Geometry chapter , the Grade 10 Trigonometry chapter or the Grade 10 Analytical Geometry chapter . This chapter revises:

1. Terminology: vertices, sides, angles, parallel lines, perpendicular lines, diagonals, bisectors, transversals
2. Properties of triangles
3. Congruence
4. Classification of angles into acute, right, obtuse, straight, reflex or revolution
5. Theorem of Pythagoras which is used to calculate the lengths of sides of a right-angled triangle

Points and lines

The two simplest objects in geometry are points and lines .

A point is a coordinate that marks a position in space (on a number line, on a plane or in three dimensions or even more) and is denoted by a dot. Points are usually labelled with a capital letter. Some examples of how points can be represented are shown in [link] .

A line is a continuous set of coordinates in space and can be thought of as being formed when many points are placed next to each other. Lines can be straight or curved, but are always continuous. This means that there are never any breaks in the lines (if there are, they would be distinct lines denoted separately). The endpoints of lines are labeled with capital letters. Examples of two lines are shown in [link] .

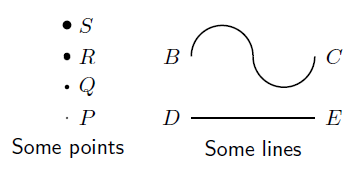
Lines are labelled according to the start point and end point. We call the line that starts at a point $A$ and ends at a point $B$ , $AB$ . Since the line from point $B$ to point $A$ is the same as the line from point $A$ to point $B$ , we have that $AB=BA$ .

When there is no ambiguity (which is the case throughout this text) the length of the line between points $A$ and $B$ is also denoted $AB$ , the same as the notation to refer to the line itself. So if we say $AB=CD$ we mean that the length of the line between $A$ and $B$ is equal to the length of the line between $C$ and $D$ .

Note: in higher mathematics, where there might be some ambiguity between when we want refer to the length of the line and when we just want to refer to the line itself, the notation $\left|AB\right|$ is usually used to refer to the length of the line. In this case, if one says $\left|AB\right|=\left|CD\right|$ , it means the lengths of the lines are the same, whereas if one says $AB=CD$ , it means that the two lines actually coincide (i.e. they are the same). Throughout this text, however, this notation will not be used, and $AB=CD$ ALWAYS implies that the lengths are the same.

A line is measured in units of length . Some common units of length are listed in [link] .

Angles

An angle is formed when two straight lines meet at a point. The point at which two lines meet is known as a vertex . Angles are labelled with a $\widehat{}$ called a caret on a letter. For example, in [link] the angle is at $\widehat{B}$ . Angles can also be labelled according to the line segments that make up the angle. For example, in [link] the angle is made up when line segments $CB$ and $BA$ meet. So, the angle can be referred to as $\angle CBA$ or $\angle ABC$ or, if there is no ambiguity (i.e. there is only one angle at $B$ ) sometimes simply $\angle B$ . The $\angle$ symbol is a short method of writing angle in geometry.