| << Chapter < Page | Chapter >> Page > |
Consider the quadratic function Find the zeros of Does the parabola open upward or downward?
The zeros are The parabola opens upward.
A large variety of real-world situations can be described using mathematical models . A mathematical model is a method of simulating real-life situations with mathematical equations. Physicists, engineers, economists, and other researchers develop models by combining observation with quantitative data to develop equations, functions, graphs, and other mathematical tools to describe the behavior of various systems accurately. Models are useful because they help predict future outcomes. Examples of mathematical models include the study of population dynamics, investigations of weather patterns, and predictions of product sales.
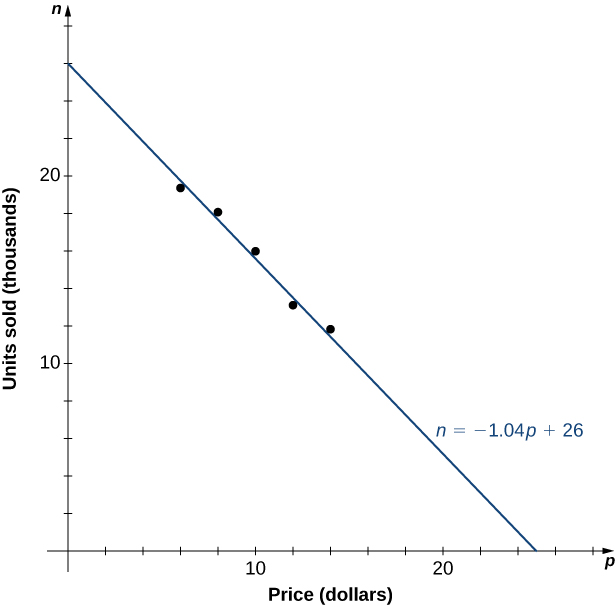
As an example, let’s consider a mathematical model that a company could use to describe its revenue for the sale of a particular item. The amount of revenue a company receives for the sale of items sold at a price of dollars per item is described by the equation The company is interested in how the sales change as the price of the item changes. Suppose the data in [link] show the number of units a company sells as a function of the price per item.
In [link] , we see the graph the number of units sold (in thousands) as a function of price (in dollars). We note from the shape of the graph that the number of units sold is likely a linear function of price per item, and the data can be closely approximated by the linear function for where predicts the number of units sold in thousands. Using this linear function, the revenue (in thousands of dollars) can be estimated by the quadratic function
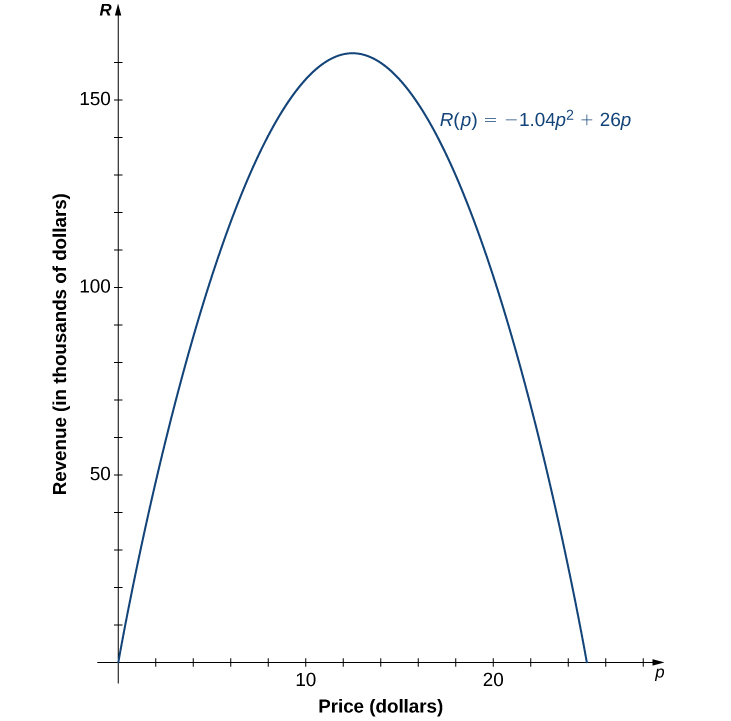
for In [link] , we use this quadratic function to predict the amount of revenue the company receives depending on the price the company charges per item. Note that we cannot conclude definitively the actual number of units sold for values of for which no data are collected. However, given the other data values and the graph shown, it seems reasonable that the number of units sold (in thousands) if the price charged is dollars may be close to the values predicted by the linear function
A company is interested in predicting the amount of revenue it will receive depending on the price it charges for a particular item. Using the data from [link] , the company arrives at the following quadratic function to model revenue as a function of price per item
for

Notification Switch
Would you like to follow the 'Calculus volume 1' conversation and receive update notifications?