8.8 Solve uniform motion and work applications  (Page 3/5)

Let’s get back to Pete and Alicia painting the room. We will let t be the number of hours it would take them to paint the room together. So in 1 hour working together they have completed $\frac{1}{t}$ of the job.

In one hour Pete did $\frac{1}{10}$ of the job. Alicia did $\frac{1}{8}$ of the job. And together they did $\frac{1}{t}$ of the job.

We can model this with the word equation and then translate to a rational equation. To find the time it would take them if they worked together, we solve for t .

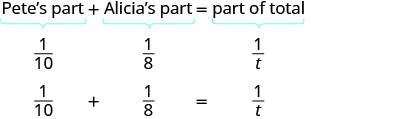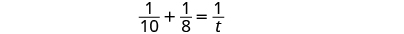Multiply by the LCD, $40t$ .
Distribute.
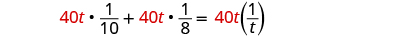Simplify and solve.
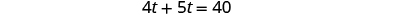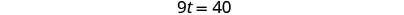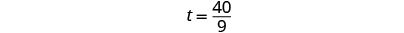We’ll write as a mixed number so that we can convert it to hours and minutes.
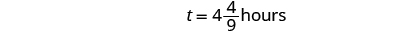Remember, 1 hour = 60 minutes.
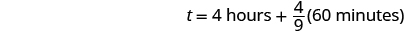Multiply, and then round to the nearest minute.
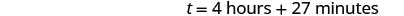	It would take Pete and Alica about 4 hours and 27 minutes to paint the room.

Keep in mind, it should take less time for two people to complete a job working together than for either person to do it alone.

The weekly gossip magazine has a big story about the Princess’ baby and the editor wants the magazine to be printed as soon as possible. She has asked the printer to run an extra printing press to get the printing done more quickly. Press #1 takes 6 hours to do the job and Press #2 takes 12 hours to do the job. How long will it take the printer to get the magazine printed with both presses running together?

Solution

This is a work problem. A chart will help us organize the information.

Let $t=$ the number of hours needed to complete the job together.
Enter the hours per job for Press #1, Press #2 and when they work together. If a job on Press #1 takes 6 hours, then in 1 hour $\frac{1}{6}$ of the job is completed. Similarly find the part of the job completed/hours for Press #2 and when they both work together.
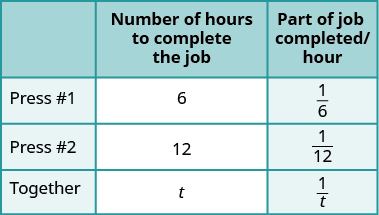Write a word sentence.
The part completed by Press #1 plus the part completed by Press #2 equals the amount completed together.
Translate to an equation.
Solve.
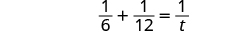Multiply by the LCD, $12t$ .
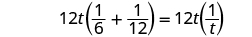Simplify.
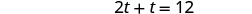	When both presses are running it takes 4 hours to do the job.

Got questions? Get instant answers now!

Got questions? Get instant answers now!

Corey can shovel all the snow from the sidewalk and driveway in 4 hours. If he and his twin Casey work together, they can finish shoveling the snow in 2 hours. How many hours would it take Casey to do the job by himself?

Solution

This is a work application. A chart will help us organize the information.
We are looking for how many hours it would take Casey to complete the job by himself.
Let $t=$ the number of hours needed for Casey to complete.
Enter the hours per job for Corey, Casey, and when they work together. If Corey takes 4 hours, then in 1 hour $\frac{1}{4}$ of the job is completed. Similarly find the part of the job completed/hours for Casey and when they both work together.
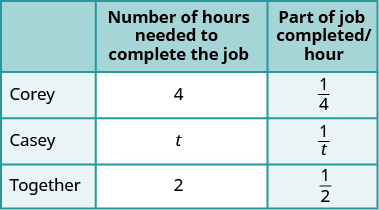Write a word sentence.
The part completed by Corey plus the part completed by Casey equals the amount completed together.
Translate to an equation:
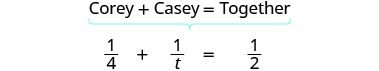Solve.
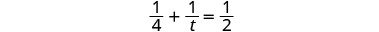Multiply by the LCD, $4t$ .
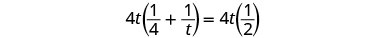Simplify.
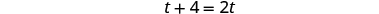	It would take Casey 4 hours to do the job alone.

Got questions? Get instant answers now!

Got questions? Get instant answers now!