| << Chapter < Page | Chapter >> Page > |
Before you get started, take this readiness quiz.
If you miss a problem, go back to the section listed and review the material.
When two quantities are related by a proportion, we say they are proportional to each other. Another way to express this relation is to talk about the variation of the two quantities. We will discuss direct variation and inverse variation in this section.
Lindsay gets paid $15 per hour at her job. If we let s be her salary and h be the number of hours she has worked, we could model this situation with the equation
Lindsay’s salary is the product of a constant, 15, and the number of hours she works. We say that Lindsay’s salary varies directly with the number of hours she works. Two variables vary directly if one is the product of a constant and the other.
For any two variables x and y , y varies directly with x if
The constant k is called the constant of variation.
In applications using direct variation, generally we will know values of one pair of the variables and will be asked to find the equation that relates x and y . Then we can use that equation to find values of y for other values of x .
If y varies directly with x and when , find the equation that relates x and y .




If varies directly as and find the equation that relates x and y .
If varies directly as and find the equation that relates x and y .
We’ll list the steps below.
Now we’ll solve a few applications of direct variation.
When Raoul runs on the treadmill at the gym, the number of calories, c , he burns varies directly with the number of minutes, m , he uses the treadmill. He burned 315 calories when he used the treadmill for 18 minutes.
ⓐ
| The number of calories,
, varies directly with
the number of minutes, , on the treadmill, and when . | |
| Write the formula for direct variation. |
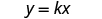 |
| We will use in place of and in place of . |
 |
| Substitute the given values for the variables. |
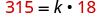 |
| Solve for the constant of variation. |
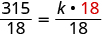 |
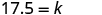 | |
| Write the equation that relates and . |
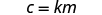 |
| Substitute in the constant of variation. |
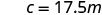 |
ⓑ
| Find when . | |
| Write the equation that relates and . |
 |
| Substitute the given value for . |
 |
| Simplify. |
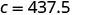 |
| Raoul would burn 437.5 calories if he used the
treadmill for 25 minutes. |
The number of calories, c , burned varies directly with the amount of time, t, spent exercising. Arnold burned 312 calories in 65 minutes exercising.
ⓐ ⓑ 432 calories

Notification Switch
Would you like to follow the 'Elementary algebra' conversation and receive update notifications?