| << Chapter < Page | Chapter >> Page > |
The population of a city is modeled by the equation where is measured in years. If the city continues to grow at this rate, how many years will it take for the population to reach one million?
about years
Find the inverse function for the exponential function
For the following exercises, use this scenario: A doctor prescribes milligrams of a therapeutic drug that decays by about each hour.
To the nearest minute, what is the half-life of the drug?
Write an exponential model representing the amount of the drug remaining in the patient’s system after hours. Then use the formula to find the amount of the drug that would remain in the patient’s system after hours. Round to the nearest hundredth of a gram.
For the following exercises, use this scenario: A soup with an internal temperature of Fahrenheit was taken off the stove to cool in a room. After fifteen minutes, the internal temperature of the soup was
Use Newton’s Law of Cooling to write a formula that models this situation.
How many minutes will it take the soup to cool to
about minutes
For the following exercises, use this scenario: The equation models the number of people in a school who have heard a rumor after days.
How many people started the rumor?
To the nearest tenth, how many days will it be before the rumor spreads to half the carrying capacity?
about days
What is the carrying capacity?
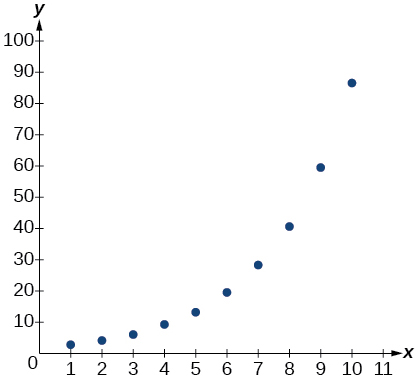
For the following exercises, enter the data from each table into a graphing calculator and graph the resulting scatter plots. Determine whether the data from the table would likely represent a function that is linear, exponential, or logarithmic.
| x | f(x) |
| 1 | 3.05 |
| 2 | 4.42 |
| 3 | 6.4 |
| 4 | 9.28 |
| 5 | 13.46 |
| 6 | 19.52 |
| 7 | 28.3 |
| 8 | 41.04 |
| 9 | 59.5 |
| 10 | 86.28 |
exponential
| x | f(x) |
| 0.5 | 18.05 |
| 1 | 17 |
| 3 | 15.33 |
| 5 | 14.55 |
| 7 | 14.04 |
| 10 | 13.5 |
| 12 | 13.22 |
| 13 | 13.1 |
| 15 | 12.88 |
| 17 | 12.69 |
| 20 | 12.45 |
Find a formula for an exponential equation that goes through the points and Then express the formula as an equivalent equation with base e.
What is the carrying capacity for a population modeled by the logistic equation What is the initial population for the model?
The population of a culture of bacteria is modeled by the logistic equation where is in days. To the nearest tenth, how many days will it take the culture to reach of its carrying capacity?
about days
For the following exercises, use a graphing utility to create a scatter diagram of the data given in the table. Observe the shape of the scatter diagram to determine whether the data is best described by an exponential, logarithmic, or logistic model. Then use the appropriate regression feature to find an equation that models the data. When necessary, round values to five decimal places.

Notification Switch
Would you like to follow the 'College algebra' conversation and receive update notifications?