7.1 Electric potential energy (Page 4/5)

University physics volume 2 Page 4 / 5

W_{2} = k \frac{q_{1} q_{2}}{r_{12}} = (9.0 \times 10^{9} \frac{N \cdot m^{2}}{C^{2}}) \frac{(2.0 \times 10^{−6} C) (3.0 \times 10^{−6} C)}{1.0 \times 10^{−2} m} = 5.4 J .

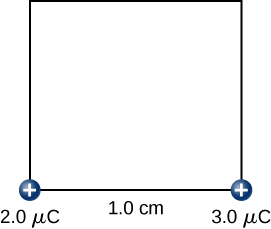
The figure shows a square with side length 1.0cm and two charges (2.0µC and 3.0µC) on adjacent corners. — Step 2. Work $W_{2}$ to bring the $+ 3.0 - μ C$ charge from infinity.

Step 3. While keeping the charges of $+ 2.0 μ C$ and $+ 3.0 μ C$ fixed in their places, bring in the $+ 4.0 - μ C$ charge to $(x, y, z) = (1.0 cm, 1.0 cm, 0)$ ( [link] ). The work done in this step is

\begin{matrix} W_{3} & = k \frac{q_{1} q_{3}}{r_{13}} + k \frac{q_{2} q_{3}}{r_{23}} \\ = (9.0 \times 10^{9} \frac{N \cdot m^{2}}{C^{2}}) [\frac{(2.0 \times 10^{−6} C) (4.0 \times 10^{−6} C)}{\sqrt{2} \times 10^{−2} m} + \frac{(3.0 \times 10^{−6} C) (4.0 \times 10^{−6} C)}{1.0 \times 10^{−2} m}] = 15.9 J . \end{matrix}

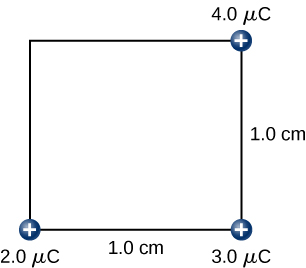
The figure shows a square with side length 1.0cm and three charges (2.0µC, 3.0µC and 4.0µC) on three corners. — Step 3. The work $W_{3}$ to bring the $+ 4.0 - μ C$ charge from infinity.

Step 4. Finally, while keeping the first three charges in their places, bring the $+ 5.0 - μ C$ charge to $(x, y, z) = (0, 1.0 cm, 0)$ ( [link] ). The work done here is

\begin{matrix} W_{4} & = k q_{4} [\frac{q_{1}}{r_{14}} + \frac{q_{2}}{r_{24}} + \frac{q_{3}}{r_{34}}], \\ = (9.0 \times 10^{9} \frac{N \cdot m^{2}}{C^{2}}) (5.0 \times 10^{−6} C) [\frac{(2.0 \times 10^{−6} C)}{1.0 \times 10^{−2} m} + \frac{(3.0 \times 10^{−6} C)}{\sqrt{2} \times 10^{−2} m} + \frac{(4.0 \times 10^{−6} C)}{1.0 \times 10^{−2} m}] = 36.5 J . \end{matrix}

The figure shows a square with side length 1.0cm and four charges (2.0µC, 3.0µC, 4.0µC and 5.0µC) located at four corners. — Step 4. The work $W_{4}$ to bring the $+ 5.0 - μ C$ charge from infinity.

Hence, the total work done by the applied force in assembling the four charges is equal to the sum of the work in bringing each charge from infinity to its final position:

W_{T} = W_{1} + W_{2} + W_{3} + W_{4} = 0 + 5.4 J + 15.9 J + 36.5 J = 57.8 J .

Significance

The work on each charge depends only on its pairwise interactions with the other charges. No more complicated interactions need to be considered; the work on the third charge only depends on its interaction with the first and second charges, the interaction between the first and second charge does not affect the third.

Check Your Understanding Is the electrical potential energy of two point charges positive or negative if the charges are of the same sign? Opposite signs? How does this relate to the work necessary to bring the charges into proximity from infinity?

positive, negative, and these quantities are the same as the work you would need to do to bring the charges in from infinity

Got questions? Get instant answers now!

Note that the electrical potential energy is positive if the two charges are of the same type, either positive or negative, and negative if the two charges are of opposite types. This makes sense if you think of the change in the potential energy $Δ U$ as you bring the two charges closer or move them farther apart. Depending on the relative types of charges, you may have to work on the system or the system would do work on you, that is, your work is either positive or negative. If you have to do positive work on the system (actually push the charges closer), then the energy of the system should increase. If you bring two positive charges or two negative charges closer, you have to do positive work on the system, which raises their potential energy. Since potential energy is proportional to 1/ r , the potential energy goes up when r goes down between two positive or two negative charges.

On the other hand, if you bring a positive and a negative charge nearer, you have to do negative work on the system (the charges are pulling you), which means that you take energy away from the system. This reduces the potential energy. Since potential energy is negative in the case of a positive and a negative charge pair, the increase in 1/ r makes the potential energy more negative, which is the same as a reduction in potential energy.

The result from [link] may be extended to systems with any arbitrary number of charges. In this case, it is most convenient to write the formula as

W_{12 \dots N} = \frac{k}{2} \sum_{i}^{N} \sum_{j}^{N} \frac{q_{i} q_{j}}{r_{i j}} for i \neq j .

The factor of 1/2 accounts for adding each pair of charges twice.

Summary

The work done to move a charge from point A to B in an electric field is path independent, and the work around a closed path is zero. Therefore, the electric field and electric force are conservative.
We can define an electric potential energy, which between point charges is $U (r) = k \frac{q Q}{r}$ , with the zero reference taken to be at infinity.
The superposition principle holds for electric potential energy; the potential energy of a system of multiple charges is the sum of the potential energies of the individual pairs.

Conceptual questions

Would electric potential energy be meaningful if the electric field were not conservative?

No. We can only define potential energies for conservative fields.

Got questions? Get instant answers now!

Why do we need to be careful about work done on the system versus work done by the system in calculations?

Got questions? Get instant answers now!

Does the order in which we assemble a system of point charges affect the total work done?

No, though certain orderings may be simpler to compute.

Got questions? Get instant answers now!

Problems

Consider a charge $Q_{1} (+ 5.0 μ C)$ fixed at a site with another charge $Q_{2}$ (charge $+ 3.0 μ C$ , mass $6.0 μ g)$ moving in the neighboring space. (a) Evaluate the potential energy of $Q_{2}$ when it is 4.0 cm from $Q_{1} .$ (b) If $Q_{2}$ starts from rest from a point 4.0 cm from $Q_{1},$ what will be its speed when it is 8.0 cm from $Q_{1}$ ? ( Note: $Q_{1}$ is held fixed in its place.)

a. $U = 3.4 J;$
b. $\frac{1}{2} m v^{2} = k Q_{1} Q_{2} (\frac{1}{r_{i}} - \frac{1}{r_{f}}) \to v = 750 m/s$

Got questions? Get instant answers now!

Two charges $Q_{1} (+ 2.00 μ C)$ and $Q_{2} (+ 2.00 μ C)$ are placed symmetrically along the x -axis at $x = \pm 3.00 cm$ . Consider a charge $Q_{3}$ of charge $+ 4.00 μ C$ and mass 10.0 mg moving along the y -axis. If $Q_{3}$ starts from rest at $y = 2.00 cm,$ what is its speed when it reaches $y = 4.00 cm?$

Got questions? Get instant answers now!

To form a hydrogen atom, a proton is fixed at a point and an electron is brought from far away to a distance of $0.529 \times 10^{−10} m,$ the average distance between proton and electron in a hydrogen atom. How much work is done?

$U = 4.36 \times 10^{−18} J$

Got questions? Get instant answers now!

(a) What is the average power output of a heart defibrillator that dissipates 400 J of energy in 10.0 ms? (b) Considering the high-power output, why doesn’t the defibrillator produce serious burns?

Got questions? Get instant answers now!

<< Chapter < Page Page > Chapter >>

Practice Key Terms 1

Read also:

Get Jobilize Job Search Mobile App in your pocket Now!

100% Free Mobile Applications
Receive real-time job alerts and never miss the right job again

Source: OpenStax, University physics volume 2. OpenStax CNX. Oct 06, 2016 Download for free at http://cnx.org/content/col12074/1.3

Google Play and the Google Play logo are trademarks of Google Inc.

Notification Switch

Would you like to follow the 'University physics volume 2' conversation and receive update notifications?

Ask

	Computer Skills Literacy MCQ By LaToya Trowers Start Quiz
	15 AP 15 Autonomic Nervous System Essay By OpenStax Start Flashcards
	NCE Ch 07 Lifestyle Career Development By Anh Dao Start Quiz
	1 Understanding Societies 1 By Jessica Collett Start Exam
	9 AP 09 Joints MCQ Quiz By OpenStax Start Quiz
	4 Sociology 04 Society and Social Interaction MCQ By OpenStax Start Quiz
	1 SCJP/OCJP Java Certification By JavaChamp Team Start Exam
	Cardiac Electrophysiology Basic By Mistry Bhavesh Start Quiz
	Anthropology Biology Culture By Richley Crapo Start Assignment
	11 AP 11 Muscular System MCQ By OpenStax Start Quiz