Coulomb's law (Page 17/2)

Siyavula textbooks: grade 11 Page 1 / 2

Introduction

In Grade 10, you learnt about the force between charges. In this chapter you will learn exactly how to determine this force andabout a basic law of electrostatics.

Forces between charges - coulomb's law

Like charges repel each other while opposite charges attract each other. If the charges are at rest then the force between them is known as the electrostatic force . The electrostatic force between charges increases when the magnitudeof the charges increases or the distance between the charges decreases.

The electrostatic force was first studied in detail by Charles Coulomb around 1784. Through his observations he was ableto show that the electrostatic force between two point-like charges is inversely proportional to the square of the distancebetween the objects. He also discovered that the force is proportional to the product of the charges on the two objects. That is:

\begin{matrix} F \propto \frac{Q_{1} Q_{2}}{r^{2}}, \end{matrix}

where $Q_{1}$ is the charge on the one point-like object, $Q_{2}$ is the charge on the second, and $r$ is the distance between the two. The magnitude of the electrostatic force between two point-likecharges is given by Coulomb's Law .

Coulomb's Law

Coulomb's Law states that the magnitude of the electrostatic force between two point charges isdirectly proportional to the magnitudes of each charge and inversely proportional to the square of the distance between thecharges:

F = k \frac{Q_{1} Q_{2}}{r^{2}}

The proportionality constant $k$ is called the electrostatic constant and has the value:

k = 8, 99 \times 10^{9} N \cdot m^{2} \cdot C^{- 2} .

Similarity of coulomb's law to newton's universal law of gravitation.

Notice how similar Coulomb's Law is to the form of Newton's Universal Law of Gravitation between two point-likeparticles:

\begin{matrix} F_{G} = G \frac{m_{1} m_{2}}{r^{2}}, \end{matrix}

where $m_{1}$ and $m_{2}$ are the masses of the two particles, $r$ is the distance between them, and $G$ is the gravitational constant.

Both laws represent the force exerted by particles (masses or charges) on each other that interact by means of a field.

Khan academy video on electrostatics - 1

Two point-like charges carrying charges of $+ 3 \times 10^{- 9} C$ and $- 5 \times 10^{- 9} C$ are $2 m$ apart. Determine the magnitude of the force between them and state whether it isattractive or repulsive.

Determine what is required
We are required to find the force between two point charges given thecharges and the distance between them.
Determine how to approach the problem
We can use Coulomb's Law to find the force.

$F = k \frac{Q_{1} Q_{2}}{r^{2}}$
Determine what is given
We are given:
- $Q_{1} = + 3 \times 10^{- 9} C$
- $Q_{2} = - 5 \times 10^{- 9} C$
- $r = 2 m$
We know that $k = 8, 99 \times 10^{9} N \cdot m^{2} \cdot C^{- 2}$ .

We can draw a diagram of the situation.
Check units
All quantities are in SI units.
Determine the magnitude of the force
Using Coulomb's Law we have

$\begin{matrix} F & = & k \frac{Q_{1} Q_{2}}{r^{2}} \\ = & (8, 99 \times 10^{9} N \cdot m^{2} / C^{2}) \frac{(3 \times 10^{- 9} C) (5 \times 10^{- 9} C)}{{(2 m)}^{2}} \\ = & 3, 37 \times 10^{- 8} N \end{matrix}$

Thus the magnitude of the force is $3, 37 \times 10^{- 8} N$ . However since both point charges have opposite signs, the force will be attractive.

Got questions? Get instant answers now!

Next is another example that demonstrates the difference in magnitude between the gravitational force and the electrostaticforce.

Determine the electrostatic force and gravitational force between two electrons $10^{- 10} m$ apart (i.e. the forces felt inside an atom).

Determine what is required
We are required to calculate the electrostatic and gravitational forces between two electrons,a given distance apart.
Determine how to approach the problem
We can use:

$F_{e} = k \frac{Q_{1} Q_{2}}{r^{2}}$

to calculate the electrostatic force and

$F_{g} = G \frac{m_{1} m_{2}}{r^{2}}$

to calculate the gravitational force.
Determine what is given
- $Q_{1} = Q_{2} = 1, 6 \times 10^{- 19} C$ (The charge on an electron)
- $m_{1} = m_{2} = 9, 1 \times 10^{- 31} kg$ (The mass of an electron)
- $r = 1 \times 10^{- 10} m$
We know that:
- $k = 8, 99 \times 10^{9} N \cdot m^{2} \cdot C^{- 2}$
- $G = 6, 67 \times 10^{- 11} N \cdot m^{2} \cdot {kg}^{- 2}$
All quantities are in SI units.

We can draw a diagram of the situation.
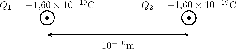
Calculate the electrostatic force
$\begin{matrix} F_{e} & = & k \frac{Q_{1} Q_{2}}{r^{2}} \\ = & (8, 99 \times 10^{9}) \frac{(- 1, 60 \times 10^{- 19}) (- 1, 60 \times 10^{- 19})}{{(10^{- 10})}^{2}} \\ = & 2, 30 \times 10^{- 8} N \end{matrix}$

Hence the magnitude of the electrostatic force between the electrons is $2, 30 \times 10^{- 8} N$ . Since electrons carry the same charge, the force is repulsive.
Calculate the gravitational force
$\begin{matrix} F_{g} & = & G \frac{m_{1} m_{2}}{r^{2}} \\ = & (6, 67 \times 10^{- 11} N \cdot m^{2} / {kg}^{2}) \frac{(9.11 \times 10^{- 31} C) (9.11 \times 10^{- 31} kg)}{{(10^{- 10} m)}^{2}} \\ = & 5, 54 \times 10^{- 51} N \end{matrix}$

The magnitude of the gravitational force between the electrons is $5, 54 \times 10^{- 51} N$ . This is an attractive force.

Notice that the gravitational force between the electrons is much smaller than the electrostatic force. For this reason, thegravitational force is usually neglected when determining the force between two charged objects.

Got questions? Get instant answers now!

<< Chapter < Page Page > Chapter >>

Read also:

Get Jobilize Job Search Mobile App in your pocket Now!

100% Free Mobile Applications
Receive real-time job alerts and never miss the right job again

Source: OpenStax, Siyavula textbooks: grade 11 physical science. OpenStax CNX. Jul 29, 2011 Download for free at http://cnx.org/content/col11241/1.2

Google Play and the Google Play logo are trademarks of Google Inc.

Notification Switch

Would you like to follow the 'Siyavula textbooks: grade 11 physical science' conversation and receive update notifications?

Ask

	Spanish Lesson 4 By Anonymous User Start Quiz
	13 AP 13 Nervous System MCQ By OpenStax Start Quiz
	How to Analyze Stocks By Yasser Ibrahim Start Quiz
	6 Enterprise JavaBeans By JavaChamp Team Start Quiz
©flickr: Gareth	Professional Etiquette MCQ By Abby Sharp Start Quiz
	44 Biology 44 Ecology and the Biosphere MCQ By OpenStax Start Quiz
	General Chemistry I MCQ By Joanna Smithback Start Quiz
	SCJP Online Exam 310-065 By Prateek Ashtikar Start Quiz
	14 AP 14 Brain Cranial Nerves MCQ By OpenStax Start Quiz
	2 Psychology MCQ 2009 1 Exam By John Gabrieli Start Exam