11.2 Newton's second law  (Page 2/3)

We only look at the forces acting in a horizontal direction (left-right) and not vertical (up-down) forces. The applied force and the force of friction will be included. The force of gravity, which is a vertical force, will not be included.

We have been given:

Applied force F ${}_1$ = 32 N

Frictional force F ${}_f$ = - 7 N

Mass m = 10 kg

To calculate the acceleration of the box we will be using the equation $F_R=ma$ . Therefore:

Always draw a force diagram although the question might not ask for it. The acceleration of the whole system is given, therefore a force diagram of the whole system will be drawn. Because the two crates are seen as a unit, the force diagram will look like this:

To find the frictional force we will apply Newton's Second Law. We are given the mass (10 + 15 kg) and the acceleration (2 m $·$ s ${}^{-2}$ ). Choose the direction of motion to be the positive direction (to the right is positive).

The frictional force is 450 N opposite to the direction of motion (to the left).

To find the tension in the rope we need to look at one of the two crates on their own. Let's choose the 10 kg crate. Firstly, we need to draw a force diagram:

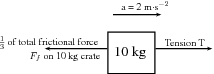
The frictional force on the 10 kg block is one third of the total, therefore:

$F_f=\frac{1}{3}\times$ 450

$F_f=150\mathrm{N}$

If we apply Newton's Second Law:

Note: If we had used the same principle and applied it to 15 kg crate, our calculations would have been the following:

The negative answer here means that the force is in the direction opposite to the motion, in other words to the left, which is correct. However, the question asks for the magnitude of the force and your answer will be quoted as 170 N.