| << Chapter < Page | Chapter >> Page > |
This is the same result that we had obtained using the concept of center of mass (COM) of the system of particles. The application of the concept of linear momentum to a system of particles, however, is useful in the expanded form, which reveals the important aspects of "internal" and "external" forces :
The right hand expression represents the vector sum of all forces on individual particles of the system.
This relation is slightly ambiguous. Left hand side symbol, " " represents net external force on the system of particles. But, the individual forces on the right hand side represent all forces i.e. both internal and external forces. This means that :
It is not difficult to resolve this apparent contradiction. Consider the example of six billiard balls, on of which is moving with certain velocity. The moving ball may collide with another ball. The two balls after collision, in turn, may collide with other balls and so on. The point is that the motions (i.e. velocity and acceleration) of the balls in this illustration are determined by the "internal" contact forces.
Momentum of a system of particles
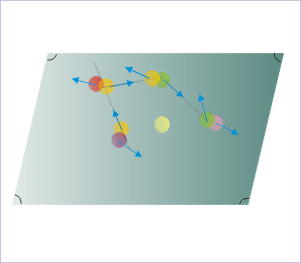
It happens (law of nature) that the motion of the "center of mass" of the system of particles depend only on the external force - even though the motion of the constituent particles depend on both "internal" and "external" forces. This is the important distinction as to the roles of external and internal forces. Internal force is not responsible for the motion of the center of mass. However, motions of the particles of the system are caused by both internal and external forces.
We again look at the process involved in the example of billiard balls. The forces arising from the collision is always a pair of forces. Actually all force exists in pair. This is the fundamental nature of force. Even the external force like force due to gravity on a projectile is one of the pair of forces. When we study projectile motion, we consider force due to gravity as the external force. We do not consider the force that the projectile applies on Earth. We think projectile as a separate system. In nutshell, we consider a single external force with respect to certain object or system and its motion.
However, the motion within a system is all inclusive i.e both pair forces are considered. It means that internal forces always appear in equal and opposite pair. The net internal force, therefore, is always zero within a system.
This is how the ambiguity in the relation above is resolved.
If no external force is involved, then change in linear momentum of the system is zero :
The relation that has resulted is for infinitesimally small change in linear momentum. By extension, a finite change in the linear momentum of the system is also zero :

Notification Switch
Would you like to follow the 'Physics for k-12' conversation and receive update notifications?