Complex numbers  (Page 16/8)

The study of mathematics continuously builds upon itself. Negative integers, for example, fill a void left by the set of positive integers. The set of rational numbers, in turn, fills a void left by the set of integers. The set of real numbers fills a void left by the set of rational numbers. Not surprisingly, the set of real numbers has voids as well. For example, we still have no solution to equations such as

Our best guesses might be +2 or –2. But if we test +2 in this equation, it does not work. If we test –2, it does not work. If we want to have a solution for this equation, we will have to go farther than we have so far. After all, to this point we have described the square root of a negative number as undefined. Fortunately, there is another system of numbers that provides solutions to problems such as these. In this section, we will explore this number system and how to work within it.

Expressing square roots of negative numbers as multiples of i

We know how to find the square root of any positive real number. In a similar way, we can find the square root of a negative number. The difference is that the root is not real. If the value in the radicand is negative, the root is said to be an imaginary number     . The imaginary number $i$ is defined as the square root of negative 1.

So, using properties of radicals,

We can write the square root of any negative number as a multiple of $i.$ Consider the square root of –25.

We use $5i$ and not $-\text{5}i$ because the principal root of $25$ is the positive root.

A complex number is the sum of a real number and an imaginary number. A complex number is expressed in standard form when written $a+bi$ where $a$ is the real part and $bi$ is the imaginary part. For example, $5+2i$ is a complex number. So, too, is $3+4\sqrt{3}i.$

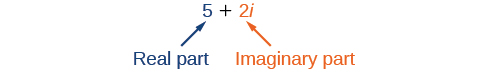
Imaginary numbers are distinguished from real numbers because a squared imaginary number produces a negative real number. Recall, when a positive real number is squared, the result is a positive real number and when a negative real number is squared, again, the result is a positive real number. Complex numbers are a combination of real and imaginary numbers.