9.7 Angular momentum and its conservation  (Page 11/7)

Calculating angular momentum of the earth

Strategy

No information is given in the statement of the problem; so we must look up pertinent data before we can calculate $L=\mathrm{I\omega }$ . First, according to [link] , the formula for the moment of inertia of a sphere is

so that

Earth’s mass $M$ is $5\text{.}\text{979}\times {\text{10}}^{\text{24}}\text{kg}$ and its radius $R$ is $6\text{.}\text{376}\times {\text{10}}^6\text{m}$ . The Earth’s angular velocity $\omega$ is, of course, exactly one revolution per day, but we must covert $\omega$ to radians per second to do the calculation in SI units.

Solution

Substituting known information into the expression for $L$ and converting $\omega$ to radians per second gives

Substituting $\mathrm{2\pi }$ rad for $1$ rev and $8\text{.}\text{64}\times {\text{10}}^4\text{s}$ for 1 day gives

Discussion

This number is large, demonstrating that Earth, as expected, has a tremendous angular momentum. The answer is approximate, because we have assumed a constant density for Earth in order to estimate its moment of inertia.

Calculating the torque putting angular momentum into a lazy susan

[link] shows a Lazy Susan food tray being rotated by a person in quest of sustenance. Suppose the person exerts a 2.50 N force perpendicular to the lazy Susan’s 0.260-m radius for 0.150 s. (a) What is the final angular momentum of the lazy Susan if it starts from rest, assuming friction is negligible? (b) What is the final angular velocity of the lazy Susan, given that its mass is 4.00 kg and assuming its moment of inertia is that of a disk?

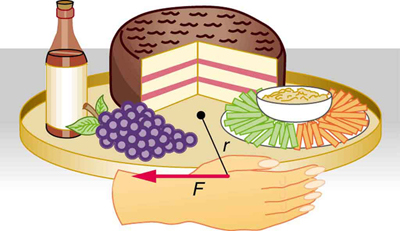
Strategy

We can find the angular momentum by solving $\text{net}\tau =\frac{\text{Δ}L}{\text{Δ}t}$ for $\text{Δ}L$ , and using the given information to calculate the torque. The final angular momentum equals the change in angular momentum, because the lazy Susan starts from rest. That is, $\text{Δ}L=L$ . To find the final velocity, we must calculate $\omega$ from the definition of $L$ in $L=\mathrm{I\omega }$ .

Solution for (a)

Solving $\text{net}\tau =\frac{\text{Δ}L}{\text{Δ}t}$ for $\text{Δ}L$ gives

Because the force is perpendicular to $r$ , we see that $\text{net}\tau =\text{rF}$ , so that

Solution for (b)

The final angular velocity can be calculated from the definition of angular momentum,

Solving for $\omega$ and substituting the formula for the moment of inertia of a disk into the resulting equation gives

And substituting known values into the preceding equation yields

Discussion

Note that the imparted angular momentum does not depend on any property of the object but only on torque and time. The final angular velocity is equivalent to one revolution in 8.71 s (determination of the time period is left as an exercise for the reader), which is about right for a lazy Susan.