7.3 Substructure of the nucleus  (Page 4/16)

The fact that nuclear forces are very strong is responsible for the very large energies emitted in nuclear decay. During decay, the forces do work, and since work is force times the distance ( $W=\text{Fd}\text{cos}\theta$ ), a large force can result in a large emitted energy. In fact, we know that there are two distinct nuclear forces because of the different types of nuclear decay—the strong nuclear force is responsible for $\alpha$ decay, while the weak nuclear force is responsible for $\beta$ decay.

The many stable and unstable nuclei we have explored, and the hundreds we have not discussed, can be arranged in a table called the chart of the nuclides    , a simplified version of which is shown in [link] . Nuclides are located on a plot of $N$ versus $Z$ . Examination of a detailed chart of the nuclides reveals patterns in the characteristics of nuclei, such as stability, abundance, and types of decay, analogous to but more complex than the systematics in the periodic table of the elements.

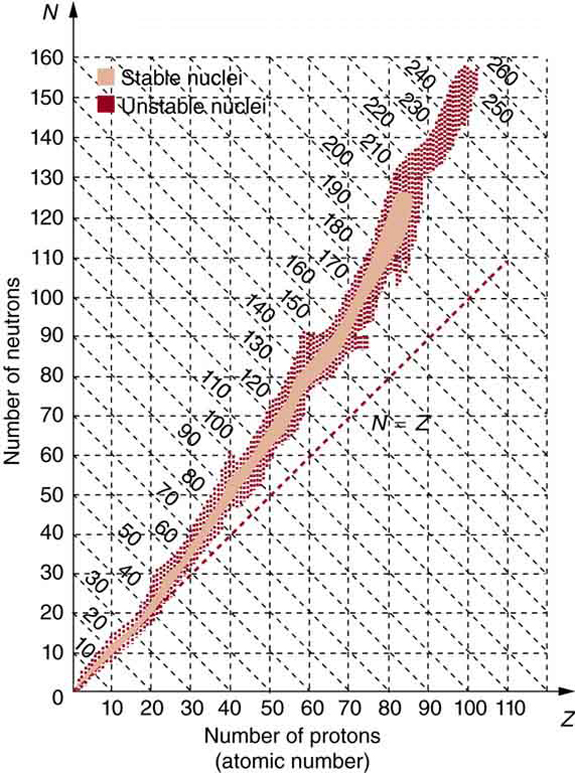
In principle, a nucleus can have any combination of protons and neutrons, but [link] shows a definite pattern for those that are stable. For low-mass nuclei, there is a strong tendency for $N$ and $Z$ to be nearly equal. This means that the nuclear force is more attractive when $N=Z$ . More detailed examination reveals greater stability when $N$ and $Z$ are even numbers—nuclear forces are more attractive when neutrons and protons are in pairs. For increasingly higher masses, there are progressively more neutrons than protons in stable nuclei. This is due to the ever-growing repulsion between protons. Since nuclear forces are short ranged, and the Coulomb force is long ranged, an excess of neutrons keeps the protons a little farther apart, reducing Coulomb repulsion. Decay modes of nuclides out of the region of stability consistently produce nuclides closer to the region of stability. There are more stable nuclei having certain numbers of protons and neutrons, called magic numbers    . Magic numbers indicate a shell structure for the nucleus in which closed shells are more stable. Nuclear shell theory has been very successful in explaining nuclear energy levels, nuclear decay, and the greater stability of nuclei with closed shells. We have been producing ever-heavier transuranic elements since the early 1940s, and we have now produced the element with $Z=\text{118}$ . There are theoretical predictions of an island of relative stability for nuclei with such high $Z$ s.

Section summary

• Two particles, both called nucleons, are found inside nuclei. The two types of nucleons are protons and neutrons; they are very similar, except that the proton is positively charged while the neutron is neutral. Some of their characteristics are given in [link] and compared with those of the electron. A mass unit convenient to atomic and nuclear processes is the unified atomic mass unit (u), defined to be
  $\mathrm{1\; u}=\text{1.6605}\times {\text{10}}^{-\text{27}}\text{kg}=\text{931.46 MeV}/c^2.$
• A nuclide is a specific combination of protons and neutrons, denoted by
  ${}_Z^A{\text{X}}_N\text{or simply}{}^A\text{X,}$
  $Z$ is the number of protons or atomic number, X is the symbol for the element, $N$ is the number of neutrons, and $A$ is the mass number or the total number of protons and neutrons,
  $A=N+Z.$
• Nuclides having the same $Z$ but different $N$ are isotopes of the same element.
• The radius of a nucleus, $r$ , is approximately
  $r=r_0{A}^{1/3},$
  where $r_0=\mathrm{1.2\; fm}$ . Nuclear volumes are proportional to $A$ . There are two nuclear forces, the weak and the strong. Systematics in nuclear stability seen on the chart of the nuclides indicate that there are shell closures in nuclei for values of $Z$ and $N$ equal to the magic numbers, which correspond to highly stable nuclei.

Conceptual questions

Problems&Exercises