| << Chapter < Page | Chapter >> Page > |
The physical principles of optical interferometry exploit the wave properties of light. Light can be thought as electromagnetic wave propagating through space. If we assume that we are dealing with a linearly polarized wave propagating in a vacuum in z direction, electric field E can be represented by a sinusoidal function of distance and time.
Where a is the amplitude of the light wave, v is the frequency, and λ is its wavelength. The term within the square brackets is called the phase of the wave. Let’s rewrite this equation in more compact form,
where is the circular frequency, and is the propagation constant. Let’s also transform this second equation into a complex exponential form,
where and is known as the complex amplitude. If n is a refractive index of a medium where the light propagates, the light wave traverses a distance d in such a medium. The equivalent optical path in this case is
When two light waves are superposed, the result intensity at any point depends on whether reinforce or cancel each other ( [link] ). This is well known phenomenon of interference. We will assume that two waves are propagating in the same direction and are polarized with their field vectors in the same plane. We will also assume that they have the same frequency. The complex amplitude at any point in the interference pattern is then the sum of the complex amplitudes of the two waves, so that we can write,
where and are the complex amplitudes of two waves. The resultant intensity is, therefore,
where and are the intensities of two waves acting separately, and is the phase difference between them. If the two waves are derived from a common source, the phase difference corresponds to an optical path difference,
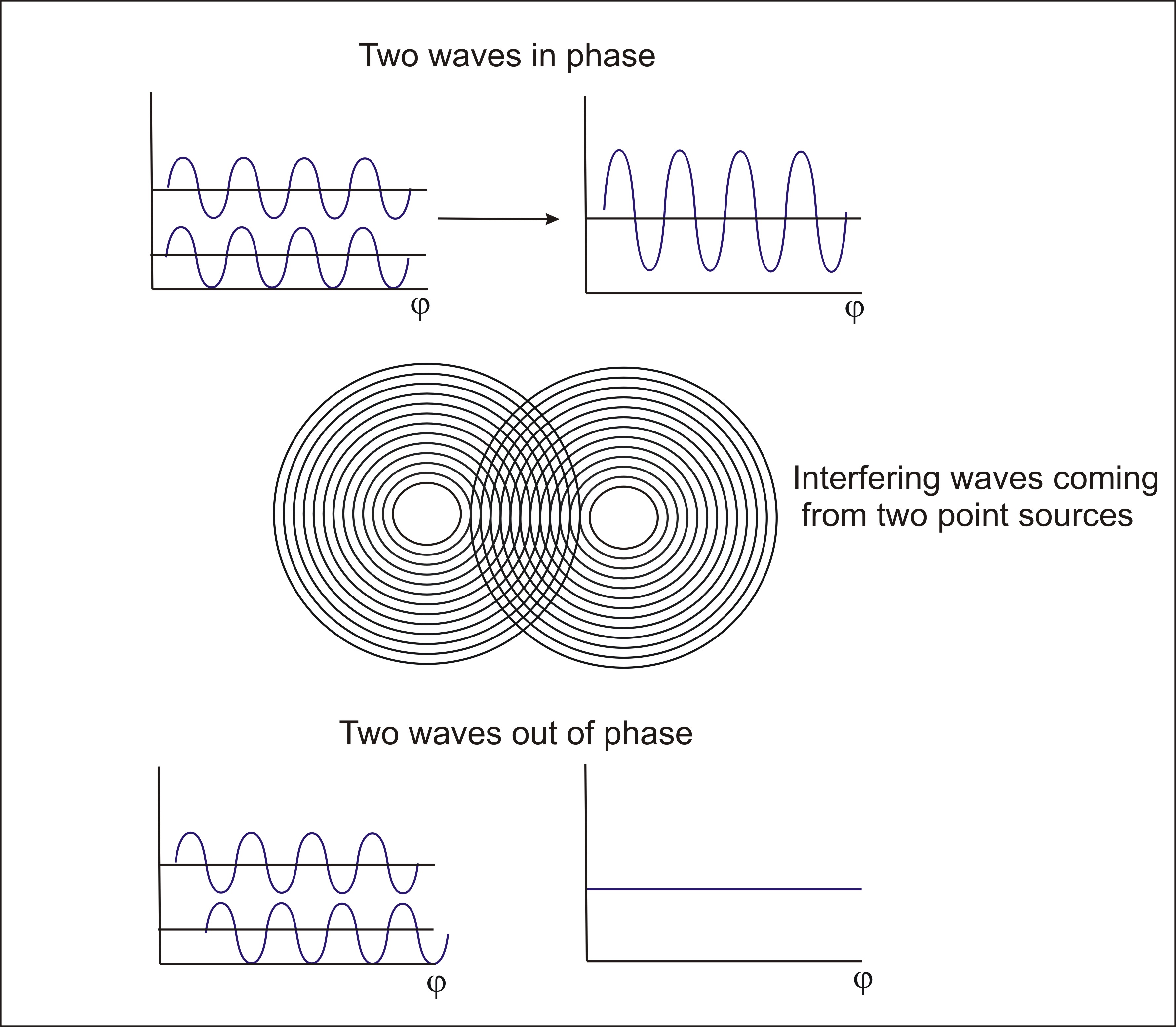
If , the phase difference between the beams, varies linearly across the field of view, the intensity varies cosinusoidally, giving rise to alternating light and dark bands or fringes ( [link] ). The intensity in an interference pattern has its maximum value
when , where m is an integer and its minimum value
when .
The principle of interferometry is widely used to develop many types of interferometric set ups. One of the earliest set ups is Michelson interferometry. The idea of this interferometry is quite simple: interference fringes are produced by splitting a beam of monochromatic light so that one beam strikes a fixed mirror and the other a movable mirror. An interference pattern results when the reflected beams are brought back together. The Michelson interferometric scheme is shown in [link] .

Notification Switch
Would you like to follow the 'Chemistry of electronic materials' conversation and receive update notifications?