4.4 Understand and use the principle of translation, learning

Mathematics

Grade 9

Quadrilaterals, perspective drawing,transformations

Module 25

Understand and use the principle of translation, learning suitable notations

Having fun with plane shapes

ACTIVITY 1

To understand and use the principle of translation, learning suitable notations

[LO 3.2, 3.7]

Transformation through translation

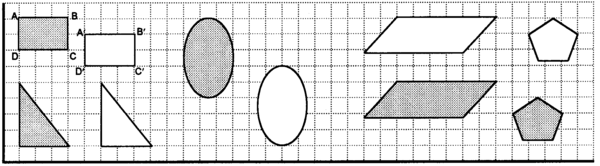
Above we have the first quadrant of a Cartesian plane . There are ten plane figures to be seen.

If you imagine that you cut out the shaded shapes above, and then move them to new positions (unshaded) by sliding them across the page, then you have translated them. Notice that they stay upright (they don’t change their orientation ). These shapes have been transformed through translation.

• Write down the names of the five shapes.

If you label the vertices of the shape, then the new position has similar (but not the same) labels. You can see this on the rectangle above. From now on, you will use the same system of labels in your work. In the rectangle, position A moves to position A, B to B, etc.

We have different ways of describing translations. This is like giving someone instructions so that they can produce the result you want.

1. For instance, if I say, “Move the oval shape 4½ units right and 3 units down,” this gives the new position of the oval.

• Describe the new position of the pentagon in the same way in words.

2. Translating the square:

Square ABCD → square ABCD means map square ABCD onto square ABCD. This is better said by specifying the positions: A (1 ; 9) → A (5 ; 8) and B(4 ; 9) → B(8 ; 8), etc.

• Use the coordinate mapping system to describe the translation of the triangle. Label the vertices A, B and C.

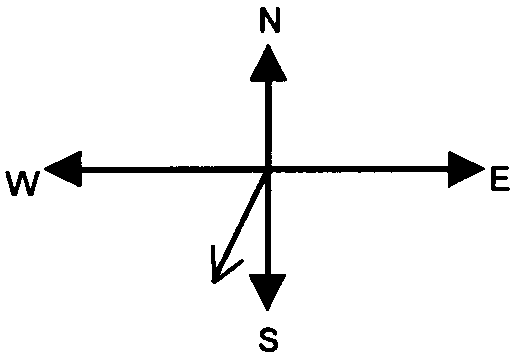
3. We can also say how far the shape must move in a certain direction, which we can specify as a compass bearing . This says how many degrees (navigators normally use three figures) clockwise we turn from due north. Refer to the figure. You can see that east is at 090° and west is at 270°. The line is at approximately 200°. The triangle above is 5 units away on a bearing of 090°. In other words, if you are at the top vertex of the triangle, you can see the new position of the top vertex 5 units away if you look east.

• Use distance and bearing to translate the parallelogram above.

• Give the shapes below (A to E) their proper names, label their vertices, and then draw them on this grid, translated to their new positions according to the descriptions below. Finally label the “new” vertices properly. Hint: work in pencil until you are sure!

  

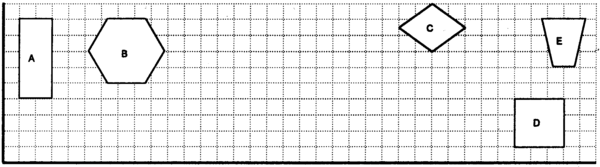
A 21 units right and 3 units down

B 11 units on a bearing of 090°

C 20 units left and 6 units down

D (31 ; 4) → (11 ; 6), (34 ; 4) → (14 ; 6), (31 ; 1) → (11 ; 3) and (34 ; 1) → (14 ; 3)

E 7 units on a bearing of 270° followed by 4 units on a bearing of 180°

ACTIVITY 2

To understand and apply reflection

[LO 3.2, 3.7]

Transformation through reflection

Look again at the last problem (E) in the previous section. Can you see that it actually gives us two translations, one after the other? The descriptions for A and C do the same! This will happen again, as it is often the simplest way to describe a complicated transformation of a shape.