4.2 The parabola  (Page 4/2)

Functions of the form $y=a{x}^2+q$

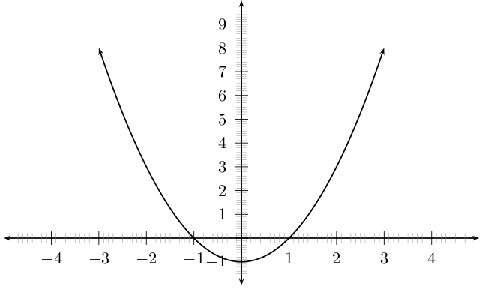
The general shape and position of the graph of the function of the form $f\left(x\right)=a{x}^2+q$ , called a parabola, is shown in [link] . These are parabolic functions.

Investigation : functions of the form $y=a{x}^2+q$

1. On the same set of axes, plot the following graphs:
   1. $a\left(x\right)=-2·x^2+1$
   2. $b\left(x\right)=-1·x^2+1$
   3. $c\left(x\right)=0·x^2+1$
   4. $d\left(x\right)=1·x^2+1$
   5. $e\left(x\right)=2·x^2+1$
   Use your results to deduce the effect of $a$ .
2. On the same set of axes, plot the following graphs:
   1. $f\left(x\right)=x^2-2$
   2. $g\left(x\right)=x^2-1$
   3. $h\left(x\right)=x^2+0$
   4. $j\left(x\right)=x^2+1$
   5. $k\left(x\right)=x^2+2$
   Use your results to deduce the effect of $q$ .

Complete the following table of values for the functions $a$ to $k$ to help with drawing the required graphs in this activity:

This simulation allows you to visualise the effect of changing a and q. Note that in this simulation q = c. Also an extra term bx has been added in. You can leave bx as 0, or you can also see what effect this has on the graph.

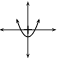
From your graphs, you should have found that $a$ affects whether the graph makes a smile or a frown. If $a<0$ , the graph makes a frown and if $a>0$ then the graph makes a smile. This is shown in [link] .

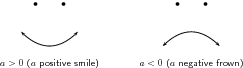
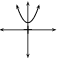
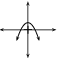
You should have also found that the value of $q$ affects whether the turning point is to the left of the $y$ -axis ( $q>0$ ) or to the right of the $y$ -axis ( $q<0$ ).

These different properties are summarised in [link] .

	$a>0$	$a<0$
$q>0$
$q<0$

Domain and range

For $f\left(x\right)=a{x}^2+q$ , the domain is $\{x:x\in \mathbb{R}\}$ because there is no value of $x\in \mathbb{R}$ for which $f\left(x\right)$ is undefined.

The range of $f\left(x\right)=a{x}^2+q$ depends on whether the value for $a$ is positive or negative. We will consider these two cases separately.

If $a>0$ then we have:

This tells us that for all values of $x$ , $f\left(x\right)$ is always greater than $q$ . Therefore if $a>0$ , the range of $f\left(x\right)=a{x}^2+q$ is $\left\{f\right(x):f(x)\in [q,\infty \left)\right\}$ .

Similarly, it can be shown that if $a<0$ that the range of $f\left(x\right)=a{x}^2+q$ is $\left\{f\right(x):f(x)\in (-\infty ,q\left]\right\}$ . This is left as an exercise.

For example, the domain of $g\left(x\right)=x^2+2$ is $\{x:x\in \mathbb{R}\}$ because there is no value of $x\in \mathbb{R}$ for which $g\left(x\right)$ is undefined. The range of $g\left(x\right)$ can be calculated as follows:

Therefore the range is $\left\{g\right(x):g(x)\in [2,\infty \left)\right\}$ .

Intercepts

For functions of the form, $y=a{x}^2+q$ , the details of calculating the intercepts with the $x$ and $y$ axis is given.

The $y$ -intercept is calculated as follows:

For example, the $y$ -intercept of $g\left(x\right)=x^2+2$ is given by setting $x=0$ to get:

The $x$ -intercepts are calculated as follows:

However, [link] is only valid if $-\frac{q}{a}\ge 0$ which means that either $q\le 0$ or $a<0$ . This is consistent with what we expect, since if $q>0$ and $a>0$ then $-\frac{q}{a}$ is negative and in this case the graph lies above the $x$ -axis and therefore does not intersect the $x$ -axis. If however, $q>0$ and $a<0$ , then $-\frac{q}{a}$ is positive and the graph is hat shaped and should have two $x$ -intercepts. Similarly, if $q<0$ and $a>0$ then $-\frac{q}{a}$ is also positive, and the graph should intersect with the $x$ -axis.