3.5 Normal, tension, and other examples of forces (2d)  (Page 2/6)

Tension

A tension     is a force along the length of a medium, especially a force carried by a flexible medium, such as a rope or cable. The word “tension ” comes from a Latin word meaning “to stretch.” Not coincidentally, the flexible cords that carry muscle forces to other parts of the body are called tendons . Any flexible connector, such as a string, rope, chain, wire, or cable, can exert pulls only parallel to its length; thus, a force carried by a flexible connector is a tension with direction parallel to the connector. It is important to understand that tension is a pull in a connector. In contrast, consider the phrase: “You can’t push a rope.” The tension force pulls outward along the two ends of a rope.

Consider a person holding a mass on a rope as shown in [link] .

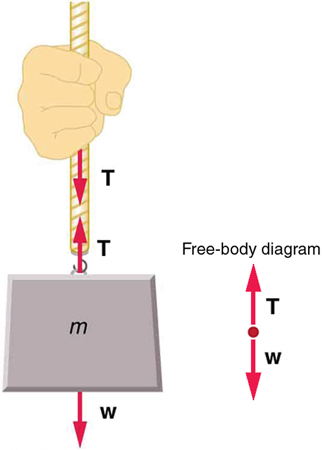
Tension in the rope must equal the weight of the supported mass, as we can prove using Newton’s second law. If the 5.00-kg mass in the figure is stationary, then its acceleration is zero, and thus ${\mathbf{\text{F}}}_{\text{net}}=0$ . The only external forces acting on the mass are its weight $\mathbf{\text{w}}$ and the tension $\mathbf{\text{T}}$ supplied by the rope. Thus,

where $T$ and $w$ are the magnitudes of the tension and weight and their signs indicate direction, with up being positive here. Thus, just as you would expect, the tension equals the weight of the supported mass:

For a 5.00-kg mass, then (neglecting the mass of the rope) we see that

If we cut the rope and insert a spring, the spring would extend a length corresponding to a force of 49.0 N, providing a direct observation and measure of the tension force in the rope.

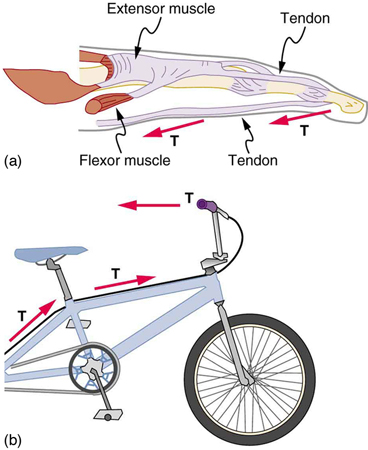
Flexible connectors are often used to transmit forces around corners, such as in a hospital traction system, a finger joint, or a bicycle brake cable. If there is no friction, the tension is transmitted undiminished. Only its direction changes, and it is always parallel to the flexible connector. This is illustrated in [link] (a) and (b).