| << Chapter < Page | Chapter >> Page > |
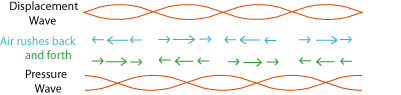
At an open end of the tube, there is nothing to stop the air rushing in and out, and so it does. What the air cannot do at the open end is build up any pressure; there is nothing for the air to build up against, and any drop in pressure will just bring air rushing in from outside the tube. So the air pressure at an open end must remain the same as the air pressure of the room. In other words, that end must have a pressure node (where the air pressure doesn't change) and (therefore) a displacement antinode.
As explained in the previous section , the standing waves in a tube must have a (displacement) node at a closed end and an antinode at an open end. In an open-open tube, this leads to a harmonic series very similar to a harmonic series produced on a string that's held at both ends. The fundamental , the lowest note possible in the tube, is the note with a wavelength twice the length of the tube (or string). The next possible note has twice the frequency (half the wavelength) of the fundamental, the next three times the frequency, the next four times, and so on.

But things are a little different for the tube that is closed at one end and open at the other. The lowest note that you might be able to get on such a tube (a fundamental that is unplayable on many instruments) has a wavelength four times the length of the tube. (You may notice that this means that a stopped tube will get a note half the frequency - an octave lower - than an open tube of the same length.) The next note that is possible on the half-closed tube has three times the frequency of the fundamental, the next five times,and so on. In other words, a stopped tube can only play the odd-numbered harmonics.


Notification Switch
Would you like to follow the 'Sound, physics and music' conversation and receive update notifications?