| << Chapter < Page | Chapter >> Page > |
The next set of fundamental identities is the set of even-odd identities. The even-odd identities relate the value of a trigonometric function at a given angle to the value of the function at the opposite angle and determine whether the identity is odd or even. (See [link] ).
| Even-Odd Identities | ||
|---|---|---|
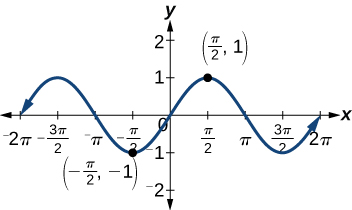
Recall that an odd function is one in which for all in the domain of The sine function is an odd function because The graph of an odd function is symmetric about the origin. For example, consider corresponding inputs of and The output of is opposite the output of Thus,
This is shown in [link] .
Recall that an even function is one in which
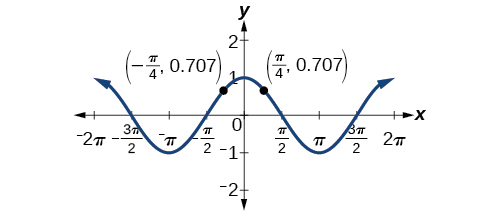
The graph of an even function is symmetric about the y- axis. The cosine function is an even function because For example, consider corresponding inputs and The output of is the same as the output of Thus,
See [link] .
For all in the domain of the sine and cosine functions, respectively, we can state the following:
The other even-odd identities follow from the even and odd nature of the sine and cosine functions. For example, consider the tangent identity, We can interpret the tangent of a negative angle as Tangent is therefore an odd function, which means that for all in the domain of the tangent function .
The cotangent identity, also follows from the sine and cosine identities. We can interpret the cotangent of a negative angle as Cotangent is therefore an odd function, which means that for all in the domain of the cotangent function .
The cosecant function is the reciprocal of the sine function, which means that the cosecant of a negative angle will be interpreted as The cosecant function is therefore odd.
Finally, the secant function is the reciprocal of the cosine function, and the secant of a negative angle is interpreted as The secant function is therefore even.
To sum up, only two of the trigonometric functions, cosine and secant, are even. The other four functions are odd, verifying the even-odd identities.
The next set of fundamental identities is the set of reciprocal identities , which, as their name implies, relate trigonometric functions that are reciprocals of each other. See [link] .

Notification Switch
Would you like to follow the 'Essential precalculus, part 2' conversation and receive update notifications?