| << Chapter < Page | Chapter >> Page > |
When describing the movement of an airplane in flight, it is important to communicate two pieces of information: the direction in which the plane is traveling and the plane’s speed. When measuring a force, such as the thrust of the plane’s engines, it is important to describe not only the strength of that force, but also the direction in which it is applied. Some quantities, such as or force, are defined in terms of both size (also called magnitude ) and direction. A quantity that has magnitude and direction is called a vector . In this text, we denote vectors by boldface letters, such as v .
A vector is a quantity that has both magnitude and direction.
A vector in a plane is represented by a directed line segment (an arrow). The endpoints of the segment are called the initial point and the terminal point of the vector. An arrow from the initial point to the terminal point indicates the direction of the vector. The length of the line segment represents its magnitude . We use the notation to denote the magnitude of the vector A vector with an initial point and terminal point that are the same is called the zero vector , denoted The zero vector is the only vector without a direction, and by convention can be considered to have any direction convenient to the problem at hand.
Vectors with the same magnitude and direction are called equivalent vectors. We treat equivalent vectors as equal, even if they have different initial points. Thus, if and are equivalent, we write
Vectors are said to be equivalent vectors if they have the same magnitude and direction.
The arrows in [link] (b) are equivalent. Each arrow has the same length and direction. A closely related concept is the idea of parallel vectors. Two vectors are said to be parallel if they have the same or opposite directions. We explore this idea in more detail later in the chapter. A vector is defined by its magnitude and direction, regardless of where its initial point is located.
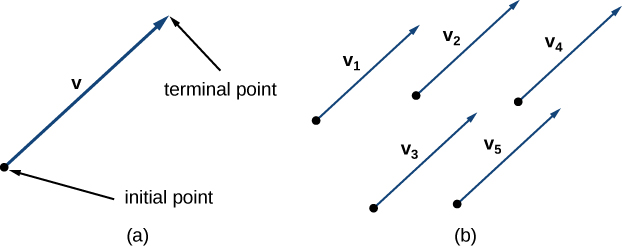
The use of boldface, lowercase letters to name vectors is a common representation in print, but there are alternative notations. When writing the name of a vector by hand, for example, it is easier to sketch an arrow over the variable than to simulate boldface type: When a vector has initial point and terminal point the notation is useful because it indicates the direction and location of the vector.
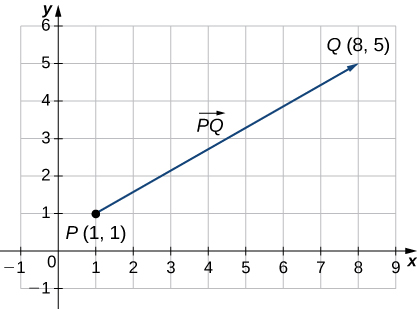
Sketch a vector in the plane from initial point to terminal point
See [link] . Because the vector goes from point to point we name it

Notification Switch
Would you like to follow the 'Calculus volume 3' conversation and receive update notifications?