| << Chapter < Page | Chapter >> Page > |
A study was done to determine the proportion of teenagers that own a car. The population proportion of teenagers that own a car is the
B: parameter
The next two questions refer to the following data:
| value | frequency |
|---|---|
| 0 | 1 |
| 1 | 4 |
| 2 | 7 |
| 3 | 9 |
| 6 | 4 |
The box plot for the data is:
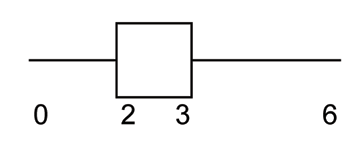
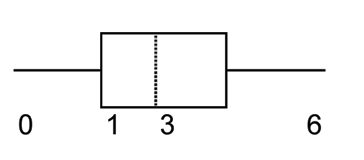

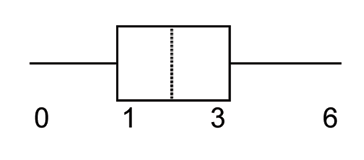
A
If 6 were added to each value of the data in the table, the 15th percentile of the new list of values is:
C: 7
The next two questions refer to the following situation:
Suppose that the probability of a drought in any independent year is 20%. Out of those years in which a drought occurs, the probability of water rationing is 10%. However, in any year, the probability of water rationing is 5%.
What is the probability of both a drought and water rationing occurring?
C: 0.02
Which of the following is true?
C: none of the above
The next two questions refer to the following situation:
Suppose that a survey yielded the following data:
| gender | apple | pumpkin | pecan |
|---|---|---|---|
| female | 40 | 10 | 30 |
| male | 20 | 30 | 10 |
Suppose that one individual is randomly chosen. The probability that the person’s favorite pie is apple or the person is male is:
D:
Suppose is: Favorite pie type and gender are independent.
The is:
A: ≈ 0
The next two questions refer to the following situation:
Let’s say that the probability that an adult watches the news at least once per week is 0.60. We randomly survey 14 people. Of interest is the number that watch the news at least once per week.
Which of the following statements is FALSE?
B: The values for are:
Find the probability that at least 6 adults watch the news.
C: 0.9417
The following histogram is most likely to be a result of sampling from which distribution?
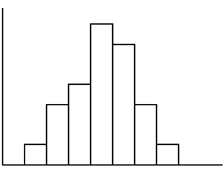
D: Binomial
The ages of campus day and evening students is known to be normally distributed. A sample of 6 campus day and evening students reported their ages (in years) as:
What is the error bound for the 90% confidence interval of the true average age?
D: 8.7
If a normally distributed random variable has and , then 97.5% of the population values lie above:
A: -1.96
The next three questions refer to the following situation:
The amount of money a customer spends in one trip to the supermarket is known to have an exponential distribution. Suppose the average amount of money a customer spends in one trip to the supermarket is $72.
What is the probability that one customer spends less than $72 in one trip to the supermarket?
A: 0.6321
How much money altogether would you expect next 5 customers to spend in one trip to the supermarket (in dollars)?
D: 360

Notification Switch
Would you like to follow the 'Collaborative statistics (with edits: teegarden)' conversation and receive update notifications?