| << Chapter < Page | Chapter >> Page > |
Other functions can also be periodic. For example, the lengths of months repeat every four years. If represents the length time, measured in years, and represents the number of days in February, then This pattern repeats over and over through time. In other words, every four years, February is guaranteed to have the same number of days as it did 4 years earlier. The positive number 4 is the smallest positive number that satisfies this condition and is called the period. A period is the shortest interval over which a function completes one full cycle—in this example, the period is 4 and represents the time it takes for us to be certain February has the same number of days.
The period of a repeating function is the number representing the interval such that for any value of
The period of the cosine, sine, secant, and cosecant functions is
The period of the tangent and cotangent functions is
Find the values of the six trigonometric functions of angle based on [link] .
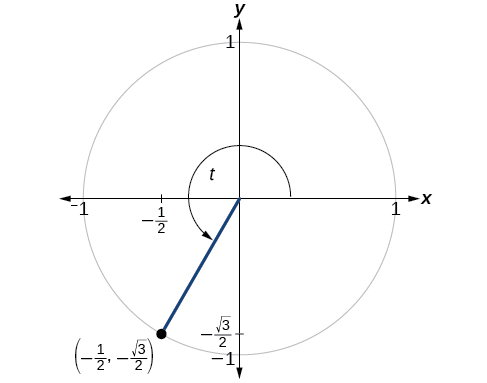
Find the values of the six trigonometric functions of angle based on [link] .
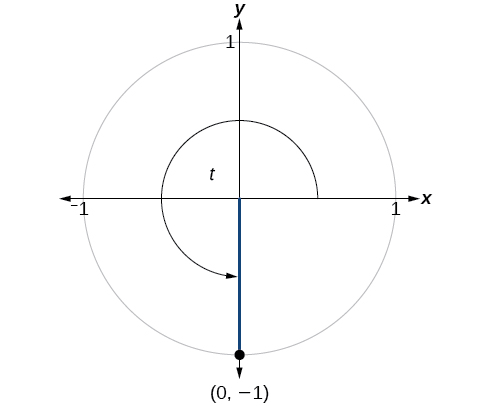
If and find
If and find
We have learned how to evaluate the six trigonometric functions for the common first-quadrant angles and to use them as reference angles for angles in other quadrants. To evaluate trigonometric functions of other angles, we use a scientific or graphing calculator or computer software. If the calculator has a degree mode and a radian mode, confirm the correct mode is chosen before making a calculation.
Evaluating a tangent function with a scientific calculator as opposed to a graphing calculator or computer algebra system is like evaluating a sine or cosine: Enter the value and press the TAN key. For the reciprocal functions, there may not be any dedicated keys that say CSC, SEC, or COT. In that case, the function must be evaluated as the reciprocal of a sine, cosine, or tangent.
If we need to work with degrees and our calculator or software does not have a degree mode, we can enter the degrees multiplied by the conversion factor to convert the degrees to radians. To find the secant of we could press
or
Given an angle measure in radians, use a scientific calculator to find the cosecant.

Notification Switch
Would you like to follow the 'Essential precalculus, part 2' conversation and receive update notifications?