| << Chapter < Page | Chapter >> Page > |
The acceleration of particle carrying charge in x-direction is :
The displacement along x-axis after time “t” is given by :
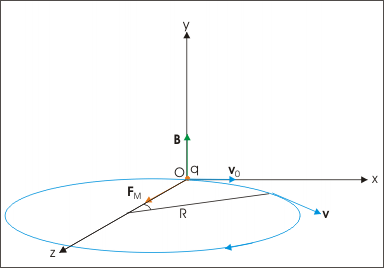
Let electric and magnetic fields align along y-direction and velocity vector is aligned along positive x-direction. Let the charge be positive and initial velocity be .In this case, velocity and magnetic field vectors are perpendicular to each other. Applying Right hand vector cross product rule, we determine that magnetic force is acting in positive z-direction. If electric field is not present, then the particle revolves along a circle in xz plane as shown in the figure below.
Motion of a charged particle in magnetic field
However, electric field in y-direction imparts acceleration in that direction. The particle, therefore, acquires velocity in y-direction and resulting motion is a helical motion. But since particle is accelerated in y –direction, the linear distance between consecutive circular elements of helix increases. In other words, the resulting motion is a helical motion with increasing pitch.
Motion of a charged particle in electric and magnetic fields
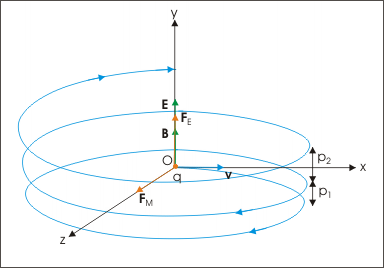
The radius of each of the circular element and other periodic attributes like time period, frequency and angular frequency are same as for the case of circular motion of charged particle in perpendicular to magnetic field.
The velocity of the particle in xz plane (as also derived in the module Motion of a charged particle in magnetic field ) is :
where α is specific charge. We know that magnetic force does not change the magnitude of velocity. It follows then that magnitude of velocity is xy plane is a constant given as :
But, there is electric field in y-direction. This imparts linear acceleration to the charged particle. As such, the particle which was initially having no component in y direction gains velocity with time as electric field imparts acceleration to the particle in y direction. The velocity components in xz plane, however, remain same. The acceleration in y-direction due to electric field is :
Since initial velocity in y-direction is zero, the velocity after time t is :
The velocity of the particle at a time t, therefore, is given in terms of component velocities as :
Component of displacement of the charged particle in xz plane is given (see module Motion of a charged particle in magnetic field ) as :
Displacement of the charged particle in xz plane
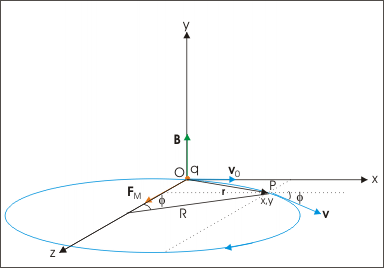
The motion in y-direction is due to electric force. Let the displacement in this direction be y after time t. Then :

Notification Switch
Would you like to follow the 'Electricity and magnetism' conversation and receive update notifications?