| << Chapter < Page | Chapter >> Page > |
where is the slope of the graph. [link] can be rewritten in a standard form
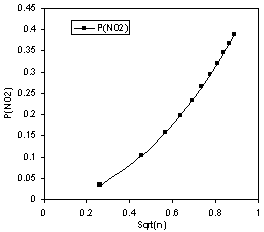
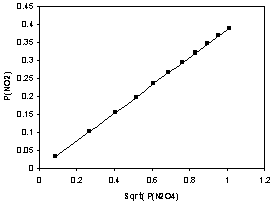
To test the accuracy of this equation and to find the value of , we return to [link] and add another column in which we calculate the value of for each of the data points. [link] makes it clear that the "constant" in [link] truly is independent of both the initial conditions and the equilibrium partial pressure of either one of the reactant orproduct. We thus refer to the constant in [link] as the reaction equilibrium constant .
| Initial | (atm) | (atm) | |
|---|---|---|---|
| 0.1 | 0.00764 | 0.0336 | 0.148 |
| 0.5 | 0.0710 | 0.102 | 0.148 |
| 1 | 0.166 | 0.156 | 0.148 |
| 1.5 | 0.267 | 0.198 | 0.148 |
| 2 | 0.371 | 0.234 | 0.148 |
| 2.5 | 0.478 | 0.266 | 0.148 |
| 3 | 0.586 | 0.294 | 0.148 |
| 3.5 | 0.695 | 0.320 | 0.148 |
| 4 | 0.805 | 0.345 | 0.148 |
| 4.5 | 0.916 | 0.368 | 0.148 |
| 5 | 1.027 | 0.389 | 0.148 |
It is very interesting to note the functional form of the equilibrium constant. The productNO 2 pressure appears in the numerator, and the exponent 2 on the pressure is the stoichiometric coefficient onNO 2 in the balanced chemical equation. The reactant N 2 O 4 pressure appears in the denominator, and the exponent 1 on the pressure is the stoichiometric coefficient onN 2 O 4 in the chemical equation.
We now investigate whether other reactions have equilibrium constants and whether the form of this equilibriumconstant is a happy coincidence or a general observation. We return to the reaction for the synthesis of ammonia .
In a previous section , we considered only the equilibrium produced when 1 mole ofN 2 is reacted with 3 moles of O 2 . We now consider a range of possible initial values of theseamounts, with the resultant equilibrium partial pressures given in [link] . In addition, anticipating the possibility of an equilibrium constant, we havecalculated the ratio of partial pressures given by:
In [link] , the equilibrium partial pressures of the gases are in a very widevariety, including whether the final pressures are greater for reactants or products. However, from the data in [link] , it is clear that, despite these variations, in [link] is essentially a constant for all of the initial conditions examined and is thus the reaction equilibrium constant for this reaction .
| V (L) | ||||||
|---|---|---|---|---|---|---|
| 10 | 1 | 3 | 0.0342 | 0.1027 | 4.82 | 6.2 × 10 5 |
| 10 | 0.1 | 0.3 | 0.0107 | 0.0322 | 0.467 | 6.0 × 10 5 |
| 100 | 0.1 | 0.3 | 0.00323 | 0.00968 | 0.0425 | 6.1 × 10 5 |
| 100 | 3 | 3 | 0.492 | 0.00880 | 0.483 | 6.1 × 10 5 |
| 100 | 1 | 3 | 0.0107 | 0.0322 | 0.467 | 6.0 × 10 5 |
| 1000 | 1.5 | 1.5 | 0.0255 | 0.00315 | 0.0223 | 6.2 × 10 5 |
Studies of many chemical reactions of gases result in the same observations. Each reaction equilibrium can bedescribed by an equilibrium constant in which the partial pressures of the products, each raised to their corresponding stoichiometriccoefficient, are multiplied together in the numerator, and the partial pressures of the reactants, each raised to theircorresponding stoichiometric coefficient, are multiplied together in the denominator. For historical reasons, this generalobservation is sometimes referred to as the Law of Mass Action .

Notification Switch
Would you like to follow the 'Concept development studies in chemistry 2012' conversation and receive update notifications?