6.1 Population growth  (Page 2/3)

The above equation can be simplified to the following.

Now, the difference between per capita birth rate and per capita death rates is further simplified by substituting the term “ r ” (per capita growth rate). So, the per capita growth rate ( r ) is equal to the per capita birth rate ( b ) minus the per capita death rate ( d ), or r = b - d .

The value “ r” can be positive, meaning the population is increasing in size; or negative, meaning the population is decreasing in size; or zero, where the population’s size is unchanging, a condition known as zero population growth. A further refinement of the formula recognizes that different species have inherent differences in their per capita growth rate (often thought of as the potential for reproduction), even under ideal conditions. Obviously, a bacterium can reproduce more rapidly and have a higher per capita growth rate than a human. The maximal growth rate for a species is its biotic potential.

Other factors that influence population growth (g)

When calculating per capita growth rate (r) as above, we are referring to a closed system where individuals from other populations are not moving in and individuals are not leaving. Immigration and emigration are two more factors that can influence the growth of a population. Immigration is the movement of individuals into a population which adds individuals to the population, and emigration is the movement of individuals out of a population which removes individuals to a population. So, the rates of immigration and emigration are two other factors population biologists need to consider when describing population growth (G).

Logistic growth

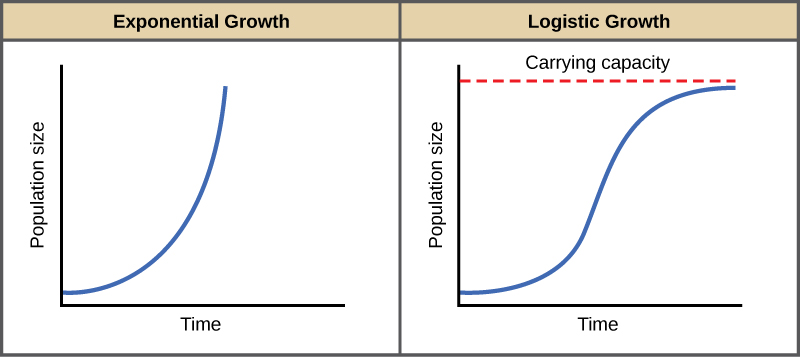
Exponential growth is possible only when natural resources are not limited. This occurs only infrequently and briefly in nature, such as when a population colonizes a new habitat or is recovering from a major disturbance. Charles Darwin recognized this fact in his description of the “struggle for existence,” which states that individuals will compete (with members of their own or other species) for limited resources. The successful ones will survive to pass on their own characteristics and traits (which we know now are transferred by genes) to the next generation at a greater rate (natural selection). To model the reality of limited resources, population ecologists developed the logistic growth model.

Carrying capacity and the logistic model

In the real world, with its limited resources, exponential growth cannot continue indefinitely. Exponential growth may occur in environments where there are few individuals and plentiful resources, but when the number of individuals gets large enough, resources will be depleted, slowing the growth rate. Eventually, the growth rate will plateau or level off ( [link] ). This population size, which represents the maximum population size that a particular environment can support, is called the carrying capacity , or K .