5.7 Non-right triangles: law of sines (Page 3/10)

Page 3 / 10

Try It

Given $α = 80°, a = 120,$ and $b = 121,$ find the missing side and angles. If there is more than one possible solution, show both.

Solution 1

\begin{array}{l} α = 80° & a = 120 \\ β \approx 83.2° & b = 121 \\ γ \approx 16.8° & c \approx 35.2 \end{array}

Solution 2

\begin{array}{l} α^{'} = 80° a^{'} = 120 \\ β^{'} \approx 96.8° b^{'} = 121 \\ γ^{'} \approx 3.2° c^{'} \approx 6.8 \end{array}

Solving for the unknown sides and angles of a ssa triangle

In the triangle shown in [link] , solve for the unknown side and angles. Round your answers to the nearest tenth.

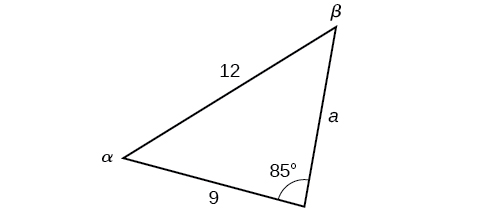
An oblique triangle with standard labels. Side b is 9, side c is 12, and angle gamma is 85. Angle alpha, angle beta, and side a are unknown.

In choosing the pair of ratios from the Law of Sines to use, look at the information given. In this case, we know the angle $γ = 85°,$ and its corresponding side $c = 12,$ and we know side $b = 9.$ We will use this proportion to solve for $β .$

\begin{array}{l} \frac{\sin (85°)}{12} = \frac{\sin β}{9} \begin{matrix}  \end{matrix} & Isolate the unknown . \\ \frac{9 \sin (85°)}{12} = \sin β \end{array}

To find $β,$ apply the inverse sine function. The inverse sine will produce a single result, but keep in mind that there may be two values for $β .$ It is important to verify the result, as there may be two viable solutions, only one solution (the usual case), or no solutions.

\begin{array}{l} β = \sin^{- 1} (\frac{9 \sin (85°)}{12}) \\ β \approx \sin^{- 1} (0.7471) \\ β \approx 48.3° \end{array}

In this case, if we subtract $β$ from 180°, we find that there may be a second possible solution. Thus, $β = 180° - 48.3° \approx 131.7° .$ To check the solution, subtract both angles, 131.7° and 85°, from 180°. This gives

α = 180° - 85° - 131.7° \approx - 36.7°,

which is impossible, and so $β \approx 48.3° .$

To find the remaining missing values, we calculate $α = 180° - 85° - 48.3° \approx 46.7° .$ Now, only side $a$ is needed. Use the Law of Sines to solve for $a$ by one of the proportions.

\begin{array}{l} \begin{array}{l} \begin{array}{l} \frac{\sin (85 °)}{12} = \frac{\sin (46.7 °)}{a} \end{array} \end{array} \\ a \frac{\sin (85 °)}{12} = \sin (46.7 °) \\ a = \frac{12 \sin (46.7 °)}{\sin (85 °)} \approx 8.8 \end{array}

The complete set of solutions for the given triangle is

\begin{array}{l} \begin{array}{l} α \approx 46.7° a \approx 8.8 \end{array} \\ β \approx 48.3° b = 9 \\ γ = 85° c = 12 \end{array}

Try It

Given $α = 80°, a = 100, b = 10,$ find the missing side and angles. If there is more than one possible solution, show both. Round your answers to the nearest tenth.

$β \approx 5.7°, γ \approx 94.3°, c \approx 101.3$

Finding the triangles that meet the given criteria

Find all possible triangles if one side has length 4 opposite an angle of 50°, and a second side has length 10.

Using the given information, we can solve for the angle opposite the side of length 10. See [link] .

\begin{array}{l} \frac{\sin α}{10} = \frac{\sin (50°)}{4} \\ \sin α = \frac{10 \sin (50°)}{4} \\ \sin α \approx 1.915 \end{array}

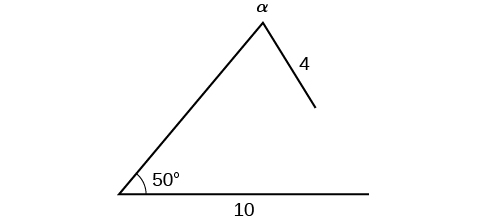
An incomplete triangle. One side has length 4 opposite a 50 degree angle, and a second side has length 10 opposite angle a. The side of length 4 is too short to reach the side of length 10, so there is no third angle.

We can stop here without finding the value of $α .$ Because the range of the sine function is $[- 1, 1],$ it is impossible for the sine value to be 1.915. In fact, inputting $\sin^{- 1} (1.915)$ in a graphing calculator generates an ERROR DOMAIN. Therefore, no triangles can be drawn with the provided dimensions.

Try It

Determine the number of triangles possible given $a = 31, b = 26, β = 48° .$

two

Finding the area of an oblique triangle using the sine function

Now that we can solve a triangle for missing values, we can use some of those values and the sine function to find the area of an oblique triangle. Recall that the area formula for a triangle is given as $Area = \frac{1}{2} b h,$ where $b$ is base and $h$ is height. For oblique triangles, we must find $h$ before we can use the area formula. Observing the two triangles in [link] , one acute and one obtuse, we can drop a perpendicular to represent the height and then apply the trigonometric property $\sin α = \frac{opposite}{hypotenuse}$ to write an equation for area in oblique triangles. In the acute triangle, we have $\sin α = \frac{h}{c}$ or $c \sin α = h .$ However, in the obtuse triangle, we drop the perpendicular outside the triangle and extend the base $b$ to form a right triangle. The angle used in calculation is $α^{'},$ or $180 - α .$

<< Chapter < Page Page > Chapter >>

Practice Key Terms 4

Read also:

Get Jobilize Job Search Mobile App in your pocket Now!

100% Free Mobile Applications
Receive real-time job alerts and never miss the right job again

Source: OpenStax, Contemporary math applications. OpenStax CNX. Dec 15, 2014 Download for free at http://legacy.cnx.org/content/col11559/1.6

Google Play and the Google Play logo are trademarks of Google Inc.

Notification Switch

Would you like to follow the 'Contemporary math applications' conversation and receive update notifications?

Ask

	How much do you love him? By Zarina Chocolate Start Quiz
	Microeconomics Test 1 By IES Portal Start Test
	Social Organization Kinship By Richley Crapo Start Assignment
	Macroeconomics MCQ By Candice Butts Start Quiz
	How to Analyze Stocks By Yasser Ibrahim Start Quiz
	Microbiology Practice Test By Sandhills MLT Start Test
	10 Neuroanatomy 10 Corticospinal Tract By Stephen Voron Start Quiz
	3 Physiotherapy Flashcards Set 3 By Rhodes Start Flashcards
	Biology Exam Final By Savannah Parrish Start Exam
	35 Biology 35 The Nervous System MCQ By OpenStax Start Quiz