10.2 Comparing two independent population proportions  (Page 2/17)

Try it

A concerned group of citizens wanted to know if the proportion of forcible rapes in Texas was different in 2011 than in 2010. Their research showed that of the 113,231 violent crimes in Texas in 2010, 7,622 of them were forcible rapes. In 2011, 7,439 of the 104,873 violent crimes were in the forcible rape category. Test at a 5% significance level. Answer the following questions:

a. Is this a test of two means or two proportions?

a. two proportions

b. Which distribution do you use to perform the test?

b. normal for two proportions

c. What is the random variable?

c. Subscripts: 1 = 2010, 2 = 2011
P ′ ₂ - P ′ ₂

d. What are the null and alternative hypothesis? Write the null and alternative hypothesis in symbols.

d. Subscripts: 1 = 2010, 2 = 2011
H ₀ : p ₁ = p ₂ H ₀ : p ₁ − p ₂ = 0
H _a : p ₁ ≠ p ₂ H _a : p ₁ − p ₂ ≠ 0

e. Is this test right-, left-, or two-tailed?

e. two-tailed

f. p -value = 0.00086

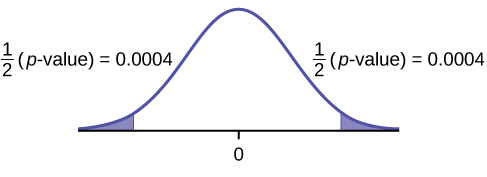
g. Do you reject or not reject the null hypothesis?

g. Reject the H ₀ .

h. At the ___ level of significance, from the sample data, there ______ (is/is not) sufficient evidence to conclude that ____________.

h. At the 5% significance level, from the sample data, there is sufficient evidence to conclude that there is a difference between the proportion of forcible rapes in 2011 and 2010.

References

Data from Educational Resources , December catalog.

Data from Hilton Hotels. Available online at http://www.hilton.com (accessed June 17, 2013).

Data from Hyatt Hotels. Available online at http://hyatt.com (accessed June 17, 2013).

Data from Statistics, United States Department of Health and Human Services.

Data from Whitney Exhibit on loan to San Jose Museum of Art.

Data from the American Cancer Society. Available online at http://www.cancer.org/index (accessed June 17, 2013).

Data from the Chancellor’s Office, California Community Colleges, November 1994.

“State of the States.” Gallup, 2013. Available online at http://www.gallup.com/poll/125066/State-States.aspx?ref=interactive (accessed June 17, 2013).

“West Nile Virus.” Centers for Disease Control and Prevention. Available online at http://www.cdc.gov/ncidod/dvbid/westnile/index.htm (accessed June 17, 2013).

Chapter review

Test of two population proportions from independent samples.

• Random variable: ${\mathrm{p\text{'}}}_A–{\mathrm{p\text{'}}}_B=$ difference between the two estimated proportions
• Distribution: normal distribution

Formula review

Pooled Proportion: p _c = $\frac{x_A+x_B}{n_A+n_B}$

Test Statistic ( z -score): $Z_c=\frac{(p^{\prime }{}_A-p^{\prime }{}_B)}{\sqrt{p_c(1-p_c)\left(\frac{1}{n_A}+\frac{1}{n_B}\right)}}$

where

$p_A^{\text{'}}$ and $p_B^{\text{'}}$ are the sample proportions, $p_A$ and $p_B$ are the population proportions,

P _c is the pooled proportion, and n _A and n _B are the sample sizes.

Use the following information for the next five exercises. Two types of phone operating system are being tested to determine if there is a difference in the proportions of system failures (crashes). Fifteen out of a random sample of 150 phones with OS ₁ had system failures within the first eight hours of operation. Nine out of another random sample of 150 phones with OS ₂ had system failures within the first eight hours of operation. OS ₂ is believed to be more stable (have fewer crashes) than OS ₁ .

Use the following information to answer the next twelve exercises. In the recent Census, three percent of the U.S. population reported being of two or more races. However, the percent varies tremendously from state to state. Suppose that two random surveys are conducted. In the first random survey, out of 1,000 North Dakotans, only nine people reported being of two or more races. In the second random survey, out of 500 Nevadans, 17 people reported being of two or more races. Conduct a hypothesis test to determine if the population percents are the same for the two states or if the percent for Nevada is statistically higher than for North Dakota.