2.1 A preview of calculus  (Page 4/13)

Estimation using rectangles

Estimate the area between the x -axis and the graph of $f\left(x\right)=x^2+1$ over the interval $\left[0,3\right]$ by using the three rectangles shown in [link] .

The areas of the three rectangles are 1 unit ² , 2 unit ² , and 5 unit ² . Using these rectangles, our area estimate is 8 unit ² .

Got questions? Get instant answers now!

Got questions? Get instant answers now!

Estimate the area between the x -axis and the graph of $f\left(x\right)=x^2+1$ over the interval $\left[0,3\right]$ by using the three rectangles shown here:

16 unit ²

Got questions? Get instant answers now!

Other aspects of calculus

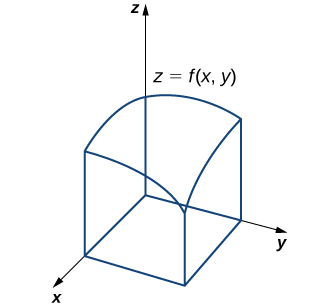
So far, we have studied functions of one variable only. Such functions can be represented visually using graphs in two dimensions; however, there is no good reason to restrict our investigation to two dimensions. Suppose, for example, that instead of determining the velocity of an object moving along a coordinate axis, we want to determine the velocity of a rock fired from a catapult at a given time, or of an airplane moving in three dimensions. We might want to graph real-value functions of two variables or determine volumes of solids of the type shown in [link] . These are only a few of the types of questions that can be asked and answered using multivariable calculus    . Informally, multivariable calculus can be characterized as the study of the calculus of functions of two or more variables. However, before exploring these and other ideas, we must first lay a foundation for the study of calculus in one variable by exploring the concept of a limit.

Key concepts

• Differential calculus arose from trying to solve the problem of determining the slope of a line tangent to a curve at a point. The slope of the tangent line indicates the rate of change of the function, also called the derivative . Calculating a derivative requires finding a limit.
• Integral calculus arose from trying to solve the problem of finding the area of a region between the graph of a function and the x -axis. We can approximate the area by dividing it into thin rectangles and summing the areas of these rectangles. This summation leads to the value of a function called the integral . The integral is also calculated by finding a limit and, in fact, is related to the derivative of a function.
• Multivariable calculus enables us to solve problems in three-dimensional space, including determining motion in space and finding volumes of solids.

Key equations

• Slope of a Secant Line
  $m_{\text{sec}}=\frac{f\left(x\right)-f\left(a\right)}{x-a}$
• Average Velocity over Interval $\left[a,t\right]$
  $v_{\text{ave}}=\frac{s\left(t\right)-s\left(a\right)}{t-a}$

For the following exercises, points $P\left(1,2\right)$ and $Q\left(x,y\right)$ are on the graph of the function $f\left(x\right)=x^2+1.$