5.7 Integrals resulting in inverse trigonometric functions  (Page 3/3)

[T] ${\displaystyle \int \frac{2e^{\mathrm{-2}x}}{\sqrt{1-e^{\mathrm{-4}x}}}dx}$ over $\left[0,2\right]$

[T] ${\displaystyle \int \frac{1}{x+x{\text{ln}}^{2}x}}$ over $\left[0,2\right]$

[T] ${\displaystyle \int \frac{{\text{sin}}^{\mathrm{-1}}x}{\sqrt{1-x^2}}}$ over $\left[\mathrm{-1},1\right]$

${\displaystyle \int \frac{e^x}{\sqrt{1-e^{2t}}}dt}$

${\displaystyle \int \frac{e^t}{1+e^{2t}}dt}$

${\displaystyle \int \frac{dt}{t\sqrt{1-{\text{ln}}^{2}t}}}$

${\displaystyle \int \frac{dt}{t\left(1+{\text{ln}}^{2}t\right)}}$

${\displaystyle \int \frac{{\text{cos}}^{\mathrm{-1}}\left(2t\right)}{\sqrt{1-4t^2}}dt}$

${\displaystyle \int \frac{e^t{\text{cos}}^{\mathrm{-1}}\left(e^t\right)}{\sqrt{1-e^{2t}}}dt}$

${\displaystyle {\int }_0^{1\text{/}2}\frac{\text{tan}\left({\text{sin}}^{\mathrm{-1}}t\right)}{\sqrt{1-t^2}}dt}$

${\displaystyle {\int }_{1\text{/}4}^{1\text{/}2}\frac{\text{tan}\left({\text{cos}}^{\mathrm{-1}}t\right)}{\sqrt{1-t^2}}dt}$

${\displaystyle {\int }_0^{1\text{/}2}\frac{\text{sin}\left({\text{tan}}^{\mathrm{-1}}t\right)}{1+t^2}dt}$

${\displaystyle {\int }_0^{1\text{/}2}\frac{\text{cos}\left({\text{tan}}^{\mathrm{-1}}t\right)}{1+t^2}dt}$

For $A>0,$ compute $I\left(A\right)={\displaystyle {\int }_{\text{−}A}^A\frac{dt}{1+t^2}}$ and evaluate $\underset{a\to \infty }{\text{lim}}I\left(A\right),$ the area under the graph of $\frac{1}{1+t^2}$ on $\left[\text{−}\infty ,\infty \right].$

For $1<B<\infty ,$ compute $I(B)={\displaystyle {\int }_1^B\frac{dt}{t\sqrt{t^2-1}}}$ and evaluate $\underset{B\to \infty }{\text{lim}}I(B),$ the area under the graph of $\frac{1}{t\sqrt{t^2-1}}$ over $[1,\infty ).$

Use the substitution $u=\sqrt{2}\text{cot}x$ and the identity $1+{\text{cot}}^{2}x={\text{csc}}^{2}x$ to evaluate ${\displaystyle \int \frac{dx}{1+{\text{cos}}^{2}x}.}$ ( Hint: Multiply the top and bottom of the integrand by ${\text{csc}}^{2}x.\text{)}$

[T] Approximate the points at which the graphs of $f(x)=2x^2-1$ and $g\left(x\right)={\left(1+4x^2\right)}^{\mathrm{-3}\text{/}2}$ intersect, and approximate the area between their graphs accurate to three decimal places.

47. [T] Approximate the points at which the graphs of $f(x)=x^2-1$ and $f(x)=x^2-1$ intersect, and approximate the area between their graphs accurate to three decimal places.

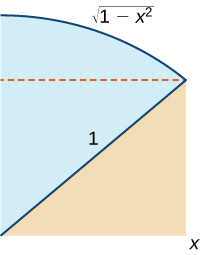
Use the following graph to prove that ${\displaystyle {\int }_0^x\sqrt{1-t^2}dt=\frac{1}{2}x\sqrt{1-x^2}+\frac{1}{2}{\text{sin}}^{\mathrm{-1}}x}.$

If $f(x)>0,f^{\prime }\text{(}x)>0$ for all $x,$ then the right-hand rule underestimates the integral ${\displaystyle {\int }_a^{b}f(x)}.$ Use a graph to justify your answer.

${\displaystyle {\int }_a^{b}f{(x)}^{2}dx=}{\displaystyle {\int }_a^{b}f(x)dx}{\displaystyle {\int }_a^{b}f(x)dx}$

If $f\left(x\right)\le g\left(x\right)$ for all $x\in \left[a,b\right],$ then ${\displaystyle {\int }_a^{b}f\left(x\right)}\le {\displaystyle {\int }_a^{b}g\left(x\right)}.$

All continuous functions have an antiderivative.

$y=3x^2-2x+1$ over $\left[\mathrm{-1},1\right]$

$y=\text{ln}\left(x^2+1\right)$ over $\left[0,e\right]$

$y=x^2\text{sin}x$ over $\left[0,\pi \right]$

$y=\sqrt{x}+\frac{1}{x}$ over $\left[1,4\right]$

${\displaystyle {\int }_{\mathrm{-1}}^1\left(x^3-2x^2+4x\right)dx}$

${\displaystyle {\int }_0^4\frac{3t}{\sqrt{1+6t^2}}dt}$

${\displaystyle {\int }_{\pi \text{/}3}^{\pi \text{/}2}2\text{sec}\left(2\theta \right)}\text{tan}\left(2\theta \right)d\theta$

${\displaystyle {\int }_0^{\pi \text{/}4}{e}^{{\text{cos}}^{2}x}}\text{sin}x\text{cos}dx$

${\displaystyle \int \frac{dx}{{\left(x+4\right)}^3}}$

${\displaystyle \int x\text{ln}\left(x^2\right)dx}$

${\displaystyle \int \frac{4x^2}{\sqrt{1-x^6}}dx}$

${\displaystyle \int \frac{e^{2x}}{1+e^{4x}}dx}$

$\frac{d}{dt}{\displaystyle {\int }_0^t\frac{\text{sin}x}{\sqrt{1+x^2}}dx}$

$\frac{d}{dx}{\displaystyle {\int }_1^{x^3}\sqrt{4-t^2}dt}$

$\frac{d}{dx}{\displaystyle {\int }_1^{\text{ln}\left(x\right)}\left(4t+e^t\right)dt}$

$\frac{d}{dx}{\displaystyle {\int }_0^{\text{cos}x}{e}^{t^2}}dt$

If the average cost per gigabyte of RAM in 2010 is $12, find the average cost per gigabyte of RAM in 1980.

The average cost per gigabyte of RAM can be approximated by the function $C\left(t\right)=8,500,000{\left(0.65\right)}^t,$ where $t$ is measured in years since 1980, and $C$ is cost in US$. Find the average cost per gigabyte of RAM for 1980 to 2010.

Find the average cost of 1GB RAM for 2005 to 2010.

The velocity of a bullet from a rifle can be approximated by $v\left(t\right)=6400t^2-6505t+2686,$ where $t$ is seconds after the shot and $v$ is the velocity measured in feet per second. This equation only models the velocity for the first half-second after the shot: $0\le t\le 0.5.$ What is the total distance the bullet travels in 0.5 sec?

What is the average velocity of the bullet for the first half-second?