| << Chapter < Page | Chapter >> Page > |
In the next example, even after distributing, we still have fractions to clear.
Solve: .
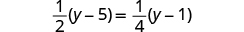 | ||
| Distribute. |
 | |
| Simplify. |
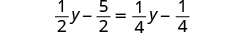 | |
| Multiply by the LCD, 4. |
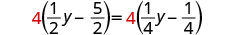 | |
| Distribute. |
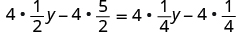 | |
| Simplify. |
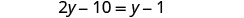 | |
| Collect the variables to the left. |
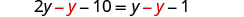 | |
| Simplify. |
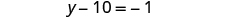 | |
| Collect the constants to the right. |
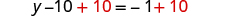 | |
| Simplify. |
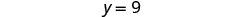 | |
| Check: |
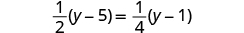 |
|
| Let . |
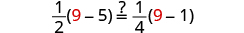 |
|
| Finish the check on your own. |
Solve: .
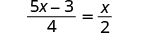 | ||
| Multiply by the LCD, 4. |
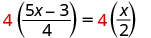 | |
| Simplify. |
 | |
| Collect the variables to the right. |
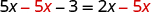 | |
| Simplify. |
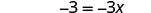 | |
| Divide. |
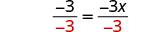 | |
| Simplify. |
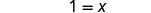 | |
| Check: |
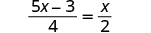 |
|
| Let . |
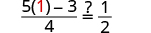 |
|
 |
||
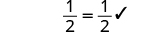 |
Solve: .
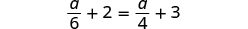 | ||
| Multiply by the LCD, 12. |
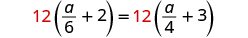 | |
| Distribute. |
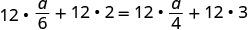 | |
| Simplify. |
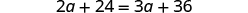 | |
| Collect the variables to the right. |
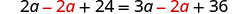 | |
| Simplify. |
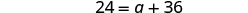 | |
| Collect the constants to the left. |
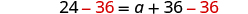 | |
| Simplify. |
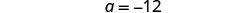 | |
| Check: |
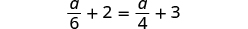 |
|
| Let . |
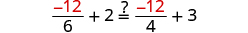 |
|
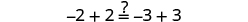 |
||
 |
Solve: .
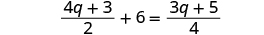 | ||
| Multiply by the LCD, 4. |
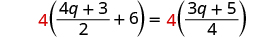 | |
| Distribute. |
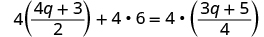 | |
| Simplify. |
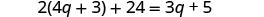

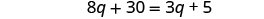 | |
| Collect the variables to the left. |
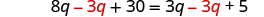 | |
| Simplify. |
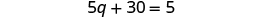 | |
| Collect the constants to the right. |
 | |
| Simplify. |
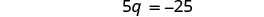 | |
| Divide by 5. |
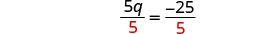 | |
| Simplify. |
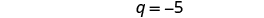 | |
| Check: |
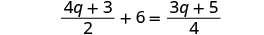 |
|
| Let . |
 |
|
| Finish the check on your own. |
Some equations have decimals in them. This kind of equation will occur when we solve problems dealing with money or percentages. But decimals can also be expressed as fractions. For example, and . So, with an equation with decimals, we can use the same method we used to clear fractions—multiply both sides of the equation by the least common denominator.
Solve: .
Look at the decimals and think of the equivalent fractions.
Notice, the LCD is 100.
By multiplying by the LCD, we will clear the decimals from the equation.
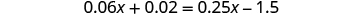 | |
| Multiply both sides by 100. |
 |
| Distribute. |
 |
| Multiply, and now we have no more decimals. |
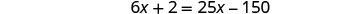 |
| Collect the variables to the right. |
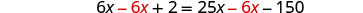 |
| Simplify. |
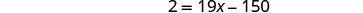 |
| Collect the constants to the left. |
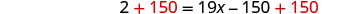 |
| Simplify. |
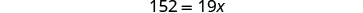 |
| Divide by 19. |
 |
| Simplify. |
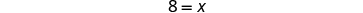 |
Check: Let
.
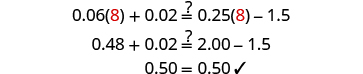 |
The next example uses an equation that is typical of the money applications in the next chapter. Notice that we distribute the decimal before we clear all the decimals.
Solve: .
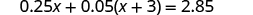 | |
| Distribute first. |
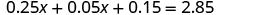 |
| Combine like terms. |
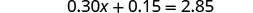 |
| To clear decimals, multiply by 100. |
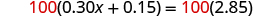 |
| Distribute. |
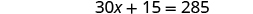 |
| Subtract 15 from both sides. |
 |
| Simplify. |
 |
| Divide by 30. |
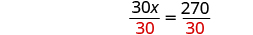 |
| Simplify. |
 |
| Check it yourself by substituting into the original equation. |
Solve Equations with Fraction Coefficients
In the following exercises, solve each equation with fraction coefficients.
Solve Equations with Decimal Coefficients
In the following exercises, solve each equation with decimal coefficients.
Coins Taylor has $2.00 in dimes and pennies. The number of pennies is 2 more than the number of dimes. Solve the equation for , the number of dimes.
Stamps Paula bought $22.82 worth of 49-cent stamps and 21-cent stamps. The number of 21-cent stamps was 8 less than the number of 49-cent stamps. Solve the equation for s , to find the number of 49-cent stamps Paula bought.
Explain how you find the least common denominator of , , and .
If an equation has several fractions, how does multiplying both sides by the LCD make it easier to solve?
Answers will vary.
If an equation has fractions only on one side, why do you have to multiply both sides of the equation by the LCD?
In the equation what is the LCD? How do you know?
100. Justifications will vary.
ⓐ After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section.
ⓑ Overall, after looking at the checklist, do you think you are well-prepared for the next section? Why or why not?

Notification Switch
Would you like to follow the 'Elementary algebra' conversation and receive update notifications?