| << Chapter < Page | Chapter >> Page > |
Since a monomial is an algebraic expression, we can use the properties of exponents to multiply monomials.
Access these online resources for additional instruction and practice with using multiplication properties of exponents:
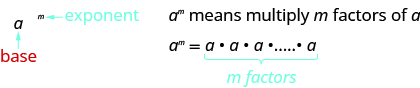
Simplify Expressions with Exponents
In the following exercises, simplify each expression with exponents.
Simplify Expressions Using the Product Property for Exponents
In the following exercises, simplify each expression using the Product Property for Exponents.
Simplify Expressions Using the Power Property for Exponents
In the following exercises, simplify each expression using the Power Property for Exponents.
Simplify Expressions Using the Product to a Power Property
In the following exercises, simplify each expression using the Product to a Power Property.
Simplify Expressions by Applying Several Properties
In the following exercises, simplify each expression.
Multiply Monomials
In the following exercises, multiply the monomials.
Mixed Practice
In the following exercises, simplify each expression.
Email Kate emails a flyer to ten of her friends and tells them to forward it to ten of their friends, who forward it to ten of their friends, and so on. The number of people who receive the email on the second round is , on the third round is , as shown in the table below. How many people will receive the email on the sixth round? Simplify the expression to show the number of people who receive the email.
| Round | Number of people |
|---|---|
| 1 | 10 |
| 2 | |
| 3 | |
| … | … |
| 6 | ? |
Salary Jamal’s boss gives him a 3% raise every year on his birthday. This means that each year, Jamal’s salary is 1.03 times his last year’s salary. If his original salary was $35,000, his salary after 1 year was , after 2 years was , after 3 years was , as shown in the table below. What will Jamal’s salary be after 10 years? Simplify the expression, to show Jamal’s salary in dollars.
| Year | Salary |
|---|---|
| 1 | |
| 2 | |
| 3 | |
| … | … |
| 10 | ? |
Clearance A department store is clearing out merchandise in order to make room for new inventory. The plan is to mark down items by 30% each week. This means that each week the cost of an item is 70% of the previous week’s cost. If the original cost of a sofa was $1,000, the cost for the first week would be and the cost of the item during the second week would be . Complete the table shown below. What will be the cost of the sofa during the fifth week? Simplify the expression, to show the cost in dollars.
| Week | Cost |
|---|---|
| 1 | |
| 2 | |
| 3 | |
| … | … |
| 8 | ? |
$168.07
Depreciation Once a new car is driven away from the dealer, it begins to lose value. Each year, a car loses 10% of its value. This means that each year the value of a car is 90% of the previous year’s value. If a new car was purchased for $20,000, the value at the end of the first year would be and the value of the car after the end of the second year would be . Complete the table shown below. What will be the value of the car at the end of the eighth year? Simplify the expression, to show the value in dollars.
| Week | Cost |
|---|---|
| 1 | |
| 2 | |
| 3 | |
| 4 | … |
| 5 | ? |
Use the Product Property for Exponents to explain why .
Answers will vary.
Explain why but .
Jorge thinks is 1. What is wrong with his reasoning?
Answers will vary.
Explain why is , and not .
ⓐ After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section.
ⓑ After reviewing this checklist, what will you do to become confident for all goals?

Notification Switch
Would you like to follow the 'Elementary algebra' conversation and receive update notifications?