1. Home
  2. Algebra and trigonometry
  3. Polynomial and rational functions
  4. Rational functions

5.6 Rational functions  (Page 9/16)

<< Chapter < Page
  Algebra and trigonometry     Page 9 / 16
Chapter >> Page >

Writing rational functions

Now that we have analyzed the equations for rational functions and how they relate to a graph of the function, we can use information given by a graph to write the function. A rational function written in factored form will have an x -intercept where each factor of the numerator is equal to zero. (An exception occurs in the case of a removable discontinuity.) As a result, we can form a numerator of a function whose graph will pass through a set of x -intercepts by introducing a corresponding set of factors. Likewise, because the function will have a vertical asymptote where each factor of the denominator is equal to zero, we can form a denominator that will produce the vertical asymptotes by introducing a corresponding set of factors.

Writing rational functions from intercepts and asymptotes

If a rational function    has x -intercepts at   x = x 1 , x 2 , ... , x n ,   vertical asymptotes at   x = v 1 , v 2 , … , v m ,   and no   x i = any  v j ,   then the function can be written in the form:

f ( x ) = a ( x − x 1 ) p 1 ( x − x 2 ) p 2 ⋯ ( x − x n ) p n ( x − v 1 ) q 1 ( x − v 2 ) q 2 ⋯ ( x − v m ) q n

where the powers   p i   or   q i   on each factor can be determined by the behavior of the graph at the corresponding intercept or asymptote, and the stretch factor   a   can be determined given a value of the function other than the x -intercept or by the horizontal asymptote if it is nonzero.

Given a graph of a rational function, write the function.

  1. Determine the factors of the numerator. Examine the behavior of the graph at the x -intercepts to determine the zeroes and their multiplicities. (This is easy to do when finding the “simplest” function with small multiplicities—such as 1 or 3—but may be difficult for larger multiplicities—such as 5 or 7, for example.)
  2. Determine the factors of the denominator. Examine the behavior on both sides of each vertical asymptote to determine the factors and their powers.
  3. Use any clear point on the graph to find the stretch factor.

Writing a rational function from intercepts and asymptotes

Write an equation for the rational function shown in [link] .

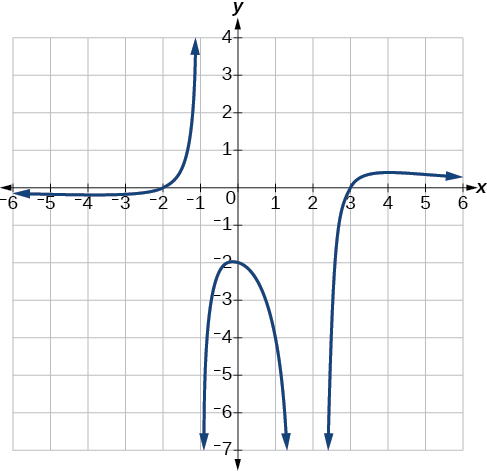
Graph of a rational function.

The graph appears to have x -intercepts at   x = –2   and   x = 3.   At both, the graph passes through the intercept, suggesting linear factors. The graph has two vertical asymptotes. The one at   x = –1   seems to exhibit the basic behavior similar to   1 x ,   with the graph heading toward positive infinity on one side and heading toward negative infinity on the other. The asymptote at   x = 2   is exhibiting a behavior similar to   1 x 2 ,   with the graph heading toward negative infinity on both sides of the asymptote. See [link] .

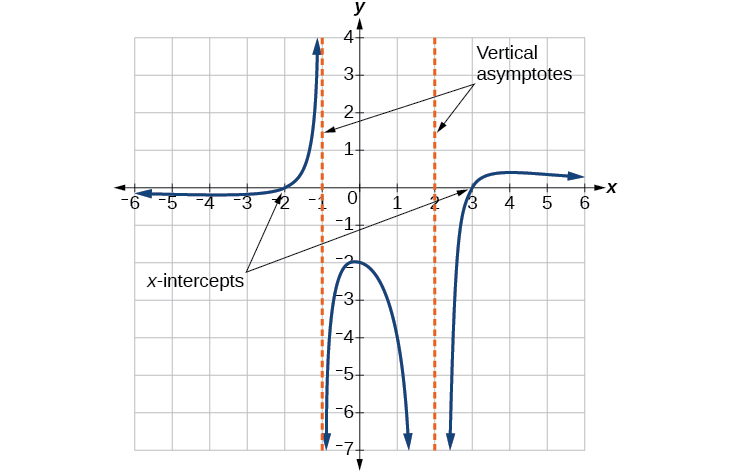
Graph of a rational function denoting its vertical asymptotes and x-intercepts.

We can use this information to write a function of the form

f ( x ) = a ( x + 2 ) ( x − 3 ) ( x + 1 ) ( x − 2 ) 2

To find the stretch factor, we can use another clear point on the graph, such as the y -intercept   ( 0 , –2 ) .

−2 = a ( 0 + 2 ) ( 0 − 3 ) ( 0 + 1 ) ( 0 − 2 ) 2 −2 = a − 6 4 a = − 8 − 6 = 4 3

This gives us a final function of   f ( x ) = 4 ( x + 2 ) ( x − 3 ) 3 ( x + 1 ) ( x − 2 ) 2 .

Got questions? Get instant answers now!
Got questions? Get instant answers now!

Access these online resources for additional instruction and practice with rational functions.
<< Chapter < Page Page > Chapter >>
Practice Key Terms 5

Read also:

Get Jobilize Job Search Mobile App in your pocket Now!

Get it on Google Play Download on the App Store Now




Source:  OpenStax, Algebra and trigonometry. OpenStax CNX. Nov 14, 2016 Download for free at https://legacy.cnx.org/content/col11758/1.6
Google Play and the Google Play logo are trademarks of Google Inc.

Notification Switch

Would you like to follow the 'Algebra and trigonometry' conversation and receive update notifications?

Ask