| << Chapter < Page | Chapter >> Page > |
A shortcut called FOIL is sometimes used to find the product of two binomials. It is called FOIL because we multiply the f irst terms, the o uter terms, the i nner terms, and then the l ast terms of each binomial.
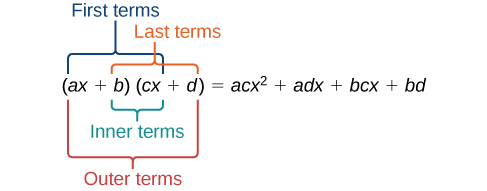
The FOIL method arises out of the distributive property. We are simply multiplying each term of the first binomial by each term of the second binomial, and then combining like terms.
Given two binomials, use FOIL to simplify the expression.
Use FOIL to find the product.
Find the product of the first terms.

Find the product of the outer terms.

Find the product of the inner terms.

Find the product of the last terms.

Certain binomial products have special forms. When a binomial is squared, the result is called a perfect square trinomial . We can find the square by multiplying the binomial by itself. However, there is a special form that each of these perfect square trinomials takes, and memorizing the form makes squaring binomials much easier and faster. Let’s look at a few perfect square trinomials to familiarize ourselves with the form.
Notice that the first term of each trinomial is the square of the first term of the binomial and, similarly, the last term of each trinomial is the square of the last term of the binomial. The middle term is double the product of the two terms. Lastly, we see that the first sign of the trinomial is the same as the sign of the binomial.
When a binomial is squared, the result is the first term squared added to double the product of both terms and the last term squared.
Given a binomial, square it using the formula for perfect square trinomials.
Expand
Begin by squaring the first term and the last term. For the middle term of the trinomial, double the product of the two terms.
Simplify
Another special product is called the difference of squares , which occurs when we multiply a binomial by another binomial with the same terms but the opposite sign. Let’s see what happens when we multiply using the FOIL method.
The middle term drops out, resulting in a difference of squares. Just as we did with the perfect squares, let’s look at a few examples.

Notification Switch
Would you like to follow the 'Algebra and trigonometry' conversation and receive update notifications?