8.2 Graphs of the other trigonometric functions (Page 5/9)

Page 5 / 9

y = A \sec (B x - C) + D

y = A \csc (B x - C) + D

a general note label

Features of the graph of y = A Sec( Bx − C )+ D

The stretching factor is $| A | .$
The period is $\frac{2 π}{| B |} .$
The domain is $x \neq \frac{C}{B} + \frac{π}{2 | B |} k,$ where $k$ is an odd integer.
The range is $(- \infty, - | A |] \cup [| A |, \infty) .$
The vertical asymptotes occur at $x = \frac{C}{B} + \frac{π}{2 | B |} k,$ where $k$ is an odd integer.
There is no amplitude.
$y = A \sec (B x)$ is an even function because cosine is an even function.

a general note label

The stretching factor is $| A | .$
The period is $\frac{2 π}{| B |} .$
The domain is $x \neq \frac{C}{B} + \frac{π}{2 | B |} k,$ where $k$ is an integer.
The range is $(- \infty, - | A |] \cup [| A |, \infty) .$
The vertical asymptotes occur at $x = \frac{C}{B} + \frac{π}{| B |} k,$ where $k$ is an integer.
There is no amplitude.
$y = A \csc (B x)$ is an odd function because sine is an odd function.

How To Feature

Given a function of the form $y = A \sec (B x),$ graph one period.

Express the function given in the form $y = A \sec (B x) .$
Identify the stretching/compressing factor, $| A | .$
Identify $B$ and determine the period, $P = \frac{2 π}{| B |} .$
Sketch the graph of $y = A \cos (B x) .$
Use the reciprocal relationship between $y = \cos x$ and $y = \sec x$ to draw the graph of $y = A \sec (B x) .$
Sketch the asymptotes.
Plot any two reference points and draw the graph through these points.

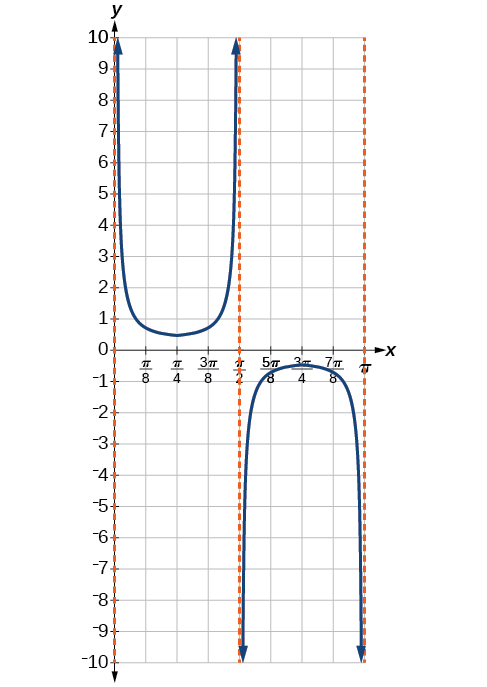
Graph one period of $f (x) = 2.5 \sec (0.4 x) .$

Step 1. The given function is already written in the general form, $y = A \sec (B x) .$
Step 2. $A = 2.5$ so the stretching factor is $2 .5 .$
Step 3. $B = 0.4$ so $P = \frac{2 π}{0.4} = 5 π .$ The period is $5 π$ units.
Step 4. Sketch the graph of the function $g (x) = 2.5 \cos (0.4 x) .$
Step 5. Use the reciprocal relationship of the cosine and secant functions to draw the cosecant function.
Steps 6–7. Sketch two asymptotes at $x = 1.25 π$ and $x = 3.75 π .$ We can use two reference points, the local minimum at $(0, 2.5)$ and the local maximum at $(2.5 π, −2.5) .$ [link] shows the graph.

Try IT Feature

Graph one period of $f (x) = - 2.5 \sec (0.4 x) .$

This is a vertical reflection of the preceding graph because $A$ is negative.

QA Feature

Do the vertical shift and stretch/compression affect the secant’s range?

Yes. The range of $f (x) = A \sec (B x - C) + D$ is $(- \infty, - | A | + D] \cup [| A | + D, \infty) .$

How To Feature

Given a function of the form $f (x) = A \sec (B x - C) + D,$ graph one period.

Express the function given in the form $y = A \sec (B x - C) + D .$
Identify the stretching/compressing factor, $| A | .$
Identify $B$ and determine the period, $\frac{2 π}{| B |} .$
Identify $C$ and determine the phase shift, $\frac{C}{B} .$
Draw the graph of $y = A \sec (B x) .$ but shift it to the right by $\frac{C}{B}$ and up by $D .$
Sketch the vertical asymptotes, which occur at $x = \frac{C}{B} + \frac{π}{2 | B |} k,$ where $k$ is an odd integer.

Graph one period of $y = 4 \sec (\frac{π}{3} x - \frac{π}{2}) + 1.$

Step 1. Express the function given in the form $y = 4 \sec (\frac{π}{3} x - \frac{π}{2}) + 1.$
Step 2. The stretching/compressing factor is $| A | = 4.$
Step 3. The period is
$\begin{array}{l} \frac{2 π}{| B |} = \frac{2 π}{\frac{π}{3}} \\ = \frac{2 π}{1} \cdot \frac{3}{π} \\ = 6 \end{array}$
Step 4. The phase shift is
$\begin{array}{l} \frac{C}{B} = \frac{\frac{π}{2}}{\frac{π}{3}} \\ = \frac{π}{2} \cdot \frac{3}{π} \\ = 1.5 \end{array}$
Step 5. Draw the graph of $y = A \sec (B x),$ but shift it to the right by $\frac{C}{B} = 1.5$ and up by $D = 6.$
Step 6. Sketch the vertical asymptotes, which occur at $x = 0, x = 3,$ and $x = 6.$ There is a local minimum at $(1.5, 5)$ and a local maximum at $(4.5, - 3) .$ [link] shows the graph.

Try IT Feature

Graph one period of $f (x) = - 6 \sec (4 x + 2) - 8.$

<< Chapter < Page Page > Chapter >>

Source: OpenStax, Algebra and trigonometry. OpenStax CNX. Nov 14, 2016 Download for free at https://legacy.cnx.org/content/col11758/1.6

Google Play and the Google Play logo are trademarks of Google Inc.

Notification Switch

Would you like to follow the 'Algebra and trigonometry' conversation and receive update notifications?

	6 Graphs of the other trigonometric functions By OpenStax Read Online Course
	4 Graphs of the other trigonometric functions By OpenStax Read Online Course
	1 Physiotherapy Flashcards Set 1 By Rhodes Start Flashcards
	7 Physiotherapy Flashcards Set 7 By Rhodes Start Flashcards
	27 Biology 27 Animal Diversity MCQ By OpenStax Start Quiz
	NCE Ch 10 Professional Orientation By Anh Dao Start Quiz
©flickr: Donnie	How well do you know Beanie Boos? By Angela Eckman Start Quiz
	Computer System Engineering By Robert Morris Start Exam
	Nutrition and Chronic Disease- Test 2 By Madison Christian Start Flashcards
	Managerial Psychology Exam By Dan Ariely Start Exam
	Social Dances 2 By Marion Cabalfin Start Quiz
	Autonomic Nervous System By Marriyam Rana Start Quiz