11.7 Probability  (Page 2/18)

Computing probabilities of equally likely outcomes

Let $S$ be a sample space for an experiment. When investigating probability, an event is any subset of $S.$ When the outcomes of an experiment are all equally likely, we can find the probability of an event by dividing the number of outcomes in the event by the total number of outcomes in $S.$ Suppose a number cube is rolled, and we are interested in finding the probability of the event “rolling a number less than or equal to 4.” There are 4 possible outcomes in the event and 6 possible outcomes in $S,$ so the probability of the event is $\frac{4}{6}=\frac{2}{3}.$

Computing the probability of the union of two events

We are often interested in finding the probability that one of multiple events occurs. Suppose we are playing a card game, and we will win if the next card drawn is either a heart or a king. We would be interested in finding the probability of the next card being a heart or a king. The union of two events     $E\text{ and }F,\text{written }E\cup F,$ is the event that occurs if either or both events occur.

Suppose the spinner in [link] is spun. We want to find the probability of spinning orange or spinning a $b.$

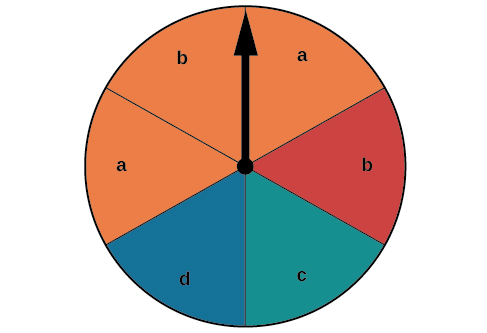
There are a total of 6 sections, and 3 of them are orange. So the probability of spinning orange is $\frac{3}{6}=\frac{1}{2}.$ There are a total of 6 sections, and 2 of them have a $b.$ So the probability of spinning a $b$ is $\frac{2}{6}=\frac{1}{3}.$ If we added these two probabilities, we would be counting the sector that is both orange and a $b$ twice. To find the probability of spinning an orange or a $b,$ we need to subtract the probability that the sector is both orange and has a $b.$

The probability of spinning orange or a $b$ is $\frac{2}{3}.$