| << Chapter < Page | Chapter >> Page > |
Consider the rational function
The function can be factored as follows:
Does this mean the function is the same as the function
The answer is no. Function does not have in its domain, but does. Graphically, we observe there is a hole in the graph of at as shown in [link] and no such hole in the graph of as shown in [link] .
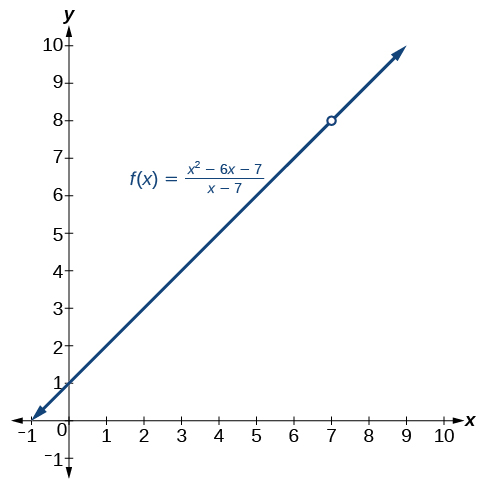
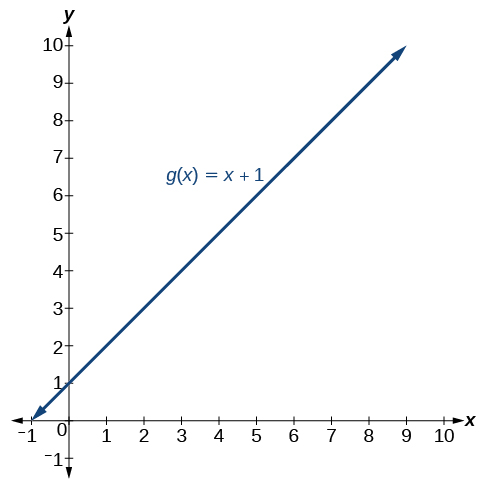
So, do these two different functions also have different limits as approaches 7?
Not necessarily. Remember, in determining a limit of a function as approaches what matters is whether the output approaches a real number as we get close to The existence of a limit does not depend on what happens when equals
Look again at [link] and [link] . Notice that in both graphs, as approaches 7, the output values approach 8. This means
Remember that when determining a limit, the concern is what occurs near not at In this section, we will use a variety of methods, such as rewriting functions by factoring, to evaluate the limit. These methods will give us formal verification for what we formerly accomplished by intuition.
Graphing a function or exploring a table of values to determine a limit can be cumbersome and time-consuming. When possible, it is more efficient to use the properties of limits , which is a collection of theorems for finding limits.
Knowing the properties of limits allows us to compute limits directly. We can add, subtract, multiply, and divide the limits of functions as if we were performing the operations on the functions themselves to find the limit of the result. Similarly, we can find the limit of a function raised to a power by raising the limit to that power. We can also find the limit of the root of a function by taking the root of the limit. Using these operations on limits, we can find the limits of more complex functions by finding the limits of their simpler component functions.
Let and represent real numbers, and and be functions, such that and For limits that exist and are finite, the properties of limits are summarized in [link]
| Constant, k | |
| Constant times a function | |
| Sum of functions | |
| Difference of functions | |
| Product of functions | |
| Quotient of functions | |
| Function raised to an exponent | where is a positive integer |
| n th root of a function, where n is a positive integer | |
| Polynomial function |

Notification Switch
Would you like to follow the 'Precalculus' conversation and receive update notifications?