2.4 Thin lenses  (Page 4/13)

Oblique parallel rays and focal plane

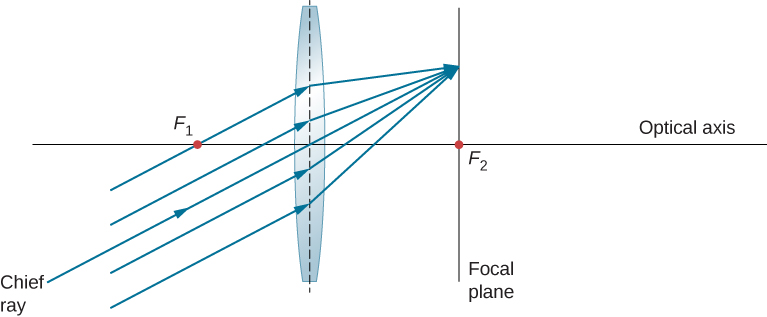
We have seen that rays parallel to the optical axis are directed to the focal point of a converging lens. In the case of a diverging lens, they come out in a direction such that they appear to be coming from the focal point on the opposite side of the lens (i.e., the side from which parallel rays enter the lens). What happens to parallel rays that are not parallel to the optical axis ( [link] )? In the case of a converging lens, these rays do not converge at the focal point. Instead, they come together on another point in the plane called the focal plane    . The focal plane contains the focal point and is perpendicular to the optical axis. As shown in the figure, parallel rays focus where the ray through the center of the lens crosses the focal plane.

Thin-lens equation

Ray tracing allows us to get a qualitative picture of image formation. To obtain numeric information, we derive a pair of equations from a geometric analysis of ray tracing for thin lenses. These equations, called the thin-lens equation and the lens maker’s equation, allow us to quantitatively analyze thin lenses.

Consider the thick bi-convex lens shown in [link] . The index of refraction of the surrounding medium is $n_1$ (if the lens is in air, then $n_1=1.00$ ) and that of the lens is $n_2$ . The radii of curvatures of the two sides are $R_1\text{and}{R}_2$ . We wish to find a relation between the object distance $d_{\text{o}}$ , the image distance $d_{\text{i}}$ , and the parameters of the lens.

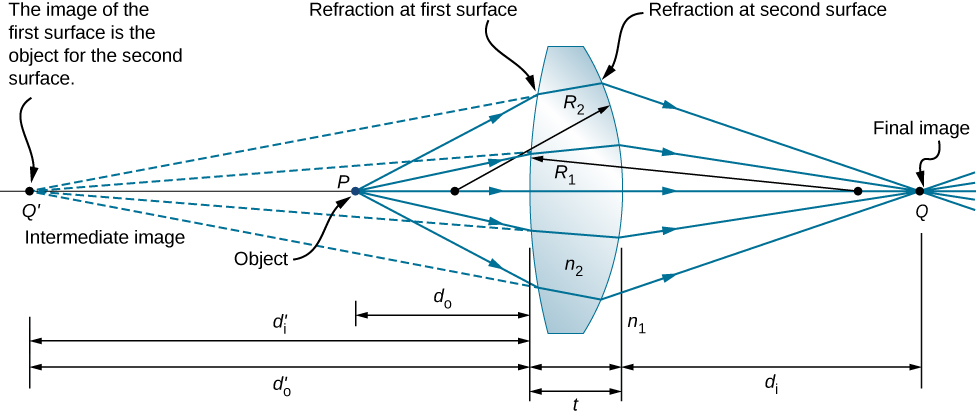
To derive the thin-lens equation , we consider the image formed by the first refracting surface (i.e., left surface) and then use this image as the object for the second refracting surface. In the figure, the image from the first refracting surface is $Q^{\prime }$ , which is formed by extending backwards the rays from inside the lens (these rays result from refraction at the first surface). This is shown by the dashed lines in the figure. Notice that this image is virtual because no rays actually pass through the point $Q^{\prime }$ . To find the image distance $d_{\text{i}}^{\prime }$ corresponding to the image $Q^{\prime }$ , we use [link] . In this case, the object distance is $d_{\text{o}}$ , the image distance is $d_{\text{i}}^{\prime }$ , and the radius of curvature is $R_1$ . Inserting these into [link] gives

The image is virtual and on the same side as the object, so $d_{\text{i}}^{\prime }<0$ and $d_{\text{o}}>0$ . The first surface is convex toward the object, so $R_1>0$ .

To find the object distance for the object Q formed by refraction from the second interface, note that the role of the indices of refraction $n_1$ and $n_2$ are interchanged in [link] . In [link] , the rays originate in the medium with index $n_2$ , whereas in [link] , the rays originate in the medium with index $n_1$ . Thus, we must interchange $n_1$ and $n_2$ in [link] . In addition, by consulting again [link] , we see that the object distance is $d_{\text{o}}^{\prime }$ and the image distance is $d_{\text{i}}$ . The radius of curvature is $R_2$ Inserting these quantities into [link] gives