12.1 Alternating current, inductance and capacitance  (Page 17/2)

Alternating current

Most students learning about electricity begin with what is known as direct current (DC), which is electricity flowing in a constant direction. DC is the kind of electricity made by a battery, with definite positive and negative terminals.

However, we have seen that the electricity produced by some generators alternates and is therefore known as alternating current (AC). The main advantage to AC is that the voltage can be changed using transformers. That means that the voltage can be stepped up at power stations to a very high voltage so that electrical energy can be transmitted along power lines at low current and therefore experience low energy loss due to heating. The voltage can then be stepped down for use in buildings and street lights.

The circuit symbol for alternating current is:

Graphs of voltage against time and current against time for an AC circuit are shown in [link]

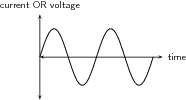
In an ideal DC circuit the current and voltage are constant. In an AC circuit the current and voltage vary with time. The value of the current or voltage at any specific time is called the instantaneous current or voltage and is calculated as follows:

$i$ is the instantaneous current. $I_{max}$ is the maximum current. $v$ is the instantaneous voltage. $V_{max}$ is the maximum voltage. $f$ is the frequency of the AC and $t$ is the time at which the instantaneous current or voltage is being calculated.

The average value we use for AC is known as the root mean square (rms) average. This is defined as:

Since AC varies sinusoidally, with as much positive as negative, doing a straight average would get you zero for the average voltage. The rms value by-passes this problem.

Exercise - alternating current

1. Explain the advantages of alternating current.
2. Write expressions for the current and voltage in an AC circuit.
3. Define the rms (root mean square) values for current and voltage for AC.
4. What is the period of the AC generated in South Africa?
5. If $V_{max}$ at a power station generator is 340 V AC, what is the mains supply (rms voltage) in our household?
6. Draw a graph of voltage vs time and current vs time for an AC circuit.

Capacitance and inductance

Capacitors and inductors are found in many circuits. Capacitors store an electric field, and are used as temporary power sources as well as to minimize power fluctuations in major circuits. Inductors work in conjunction with capacitors for electrical signal processing. Here we explain the physics and applications of both.

Capacitance

You have learnt about capacitance and capacitors in Grade 11.

In this section you will learn about capacitance in an AC circuit. A capacitor in an AC circuit has reactance . Reactance in an AC circuit plays a similar role to resistance in a DC circuit. The reactance of a capacitor $X_C$ is defined as:

where $C$ is the capacitance and $f$ is the AC frequency.

If we examine the equation for the reactance of a capacitor, we see that the frequency is in the denominator. Therefore, when the frequency is low, the capacitive reactance is very high. This is why a capacitor blocks the flow of DC and low frequency AC because its reactance increases with decreasing frequency.